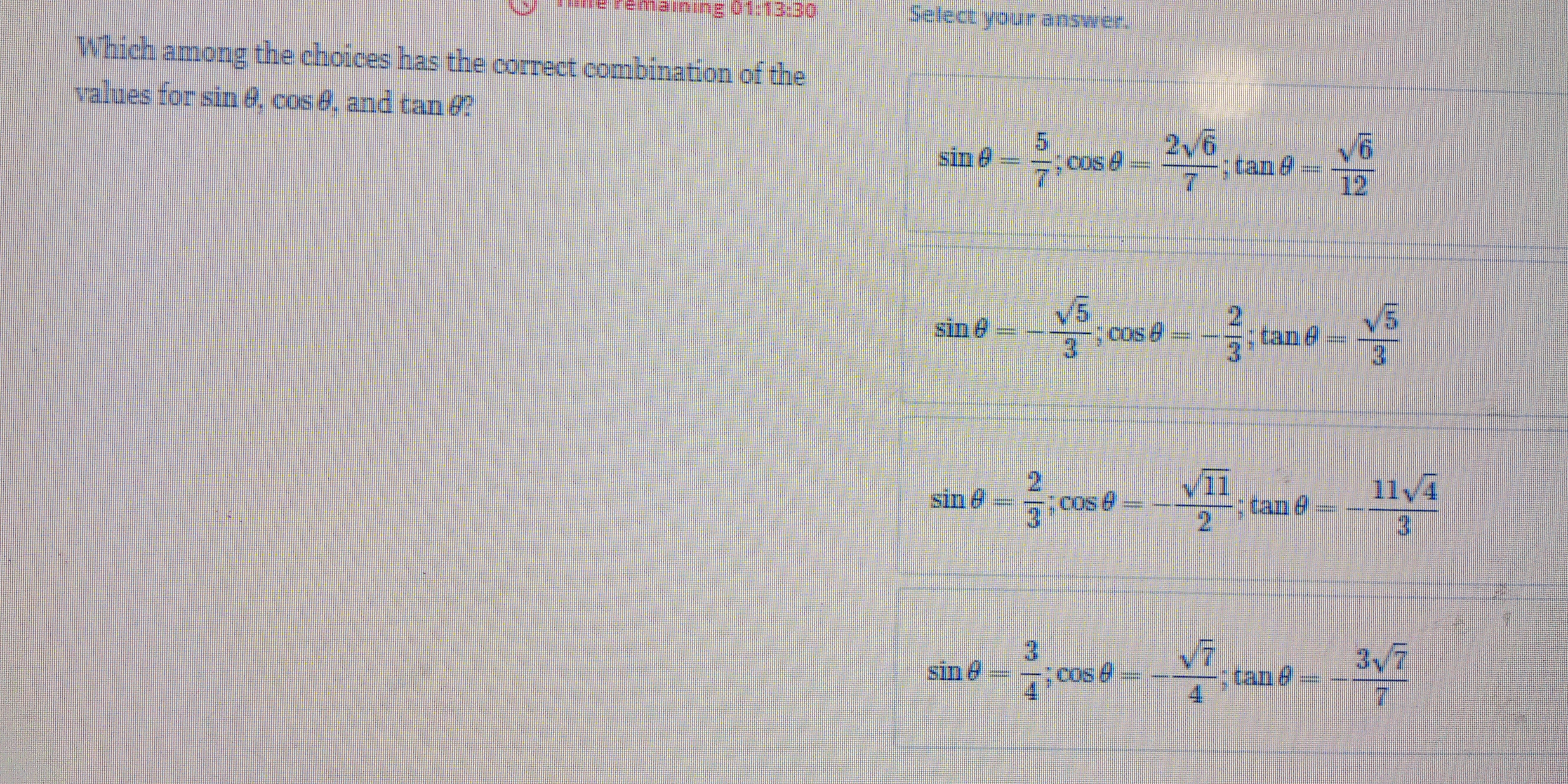
Which among the choices has the correct combination of the values for sin θ, cos θ, and tan θ?

Steps to Solve

1. Identify the Pythagorean Identity The relationship between sine, cosine, and tangent is given by the identity: $$ \sin^2 \theta + \cos^2 \theta = 1 $$ Additionally, the tangent is related as: $$ \tan \theta = \frac{\sin \theta}{\cos \theta} $$

2. Calculate for Each Option For each option, check if the values of $\sin \theta$ and $\cos \theta$ satisfy the Pythagorean identity.

   • First Option:

     • $\sin \theta = \frac{5}{7}$, $\cos \theta = -\frac{2\sqrt{6}}{7}$
     • Check: $$ \left(\frac{5}{7}\right)^2 + \left(-\frac{2\sqrt{6}}{7}\right)^2 = \frac{25}{49} + \frac{24}{49} = \frac{49}{49} = 1 $$
     • Calculate $\tan \theta$: $$ \tan \theta = \frac{\frac{5}{7}}{-\frac{2\sqrt{6}}{7}} = -\frac{5}{2\sqrt{6}} $$

   • Second Option:

     • $\sin \theta = \frac{\sqrt{5}}{3}$, $\cos \theta = -\frac{2}{3}$
     • Check: $$ \left(\frac{\sqrt{5}}{3}\right)^2 + \left(-\frac{2}{3}\right)^2 = \frac{5}{9} + \frac{4}{9} = \frac{9}{9} = 1 $$
     • Calculate $\tan \theta$: $$ \tan \theta = \frac{\frac{\sqrt{5}}{3}}{-\frac{2}{3}} = -\frac{\sqrt{5}}{2} $$

   • Third Option:

     • $\sin \theta = \frac{2}{3}$, $\cos \theta = -\frac{\sqrt{11}}{2}$
     • Check: $$ \left(\frac{2}{3}\right)^2 + \left(-\frac{\sqrt{11}}{2}\right)^2 = \frac{4}{9} + \frac{11}{4} $$
     • Compute common denominator: $$ \frac{4}{9} + \frac{99}{36} \neq 1 $$

   • Fourth Option:

     • $\sin \theta = \frac{3}{4}$, $\cos \theta = -\frac{\sqrt{7}}{4}$
     • Check: $$ \left(\frac{3}{4}\right)^2 + \left(-\frac{\sqrt{7}}{4}\right)^2 = \frac{9}{16} + \frac{7}{16} = 1 $$
     • Calculate $\tan \theta$: $$ \tan \theta = \frac{\frac{3}{4}}{-\frac{\sqrt{7}}{4}} = -\frac{3}{\sqrt{7}} $$

3. Select the Correct Option After calculations, the first, second, and fourth options satisfy the identity, and we can compare the calculated $\tan \theta$.