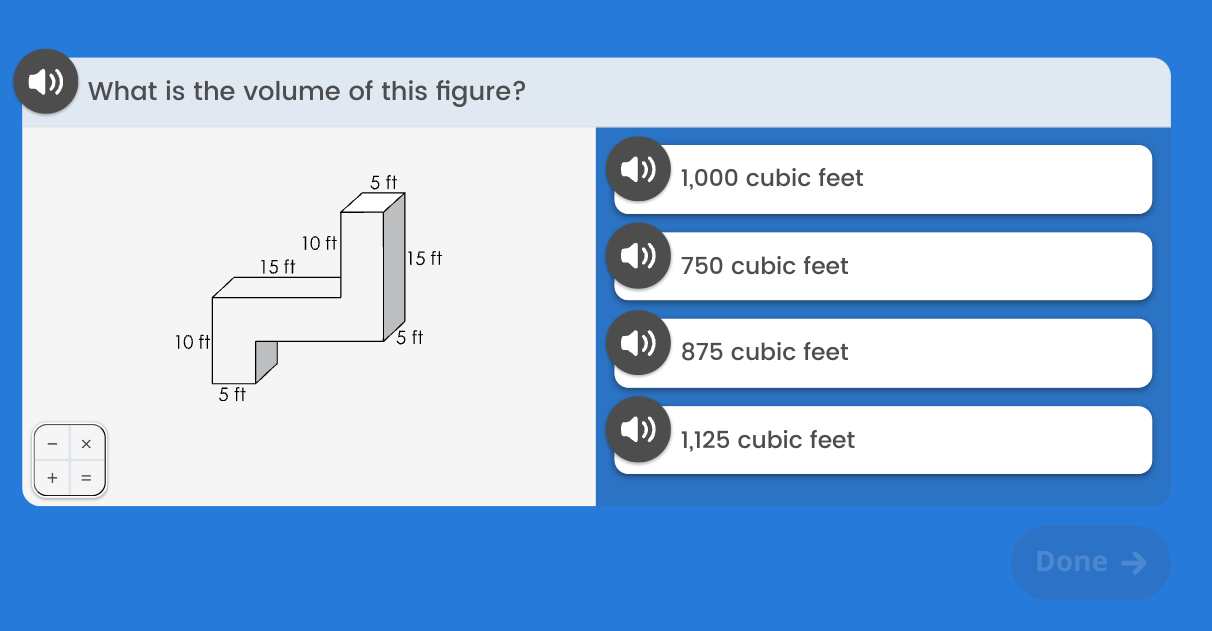
What is the volume of this figure?
Understand the Problem
The question is asking for the volume of a three-dimensional figure given its dimensions. To solve this, we need to calculate the volume using the appropriate formula based on the shape of the figure.
Answer
The volume of the figure is $1500$ cubic feet.
Answer for screen readers
The final volume of the figure is $1500$ cubic feet.
Steps to Solve
- Identify the sections of the figure
The figure can be divided into two rectangular prisms. The first prism has dimensions of 15 ft (height), 10 ft (width), and 5 ft (depth). The second prism is 15 ft (height), 5 ft (width), and 10 ft (depth).
- Calculate the volume of the first prism
Use the formula for the volume of a rectangular prism:
$$ V = \text{length} \times \text{width} \times \text{height} $$
For the first prism:
$$ V_1 = 10 , \text{ft} \times 5 , \text{ft} \times 15 , \text{ft} $$
Calculate:
$$ V_1 = 750 , \text{cubic feet} $$
- Calculate the volume of the second prism
Using the same formula for the second prism:
$$ V_2 = 5 , \text{ft} \times 10 , \text{ft} \times 15 , \text{ft} $$
Calculate:
$$ V_2 = 750 , \text{cubic feet} $$
- Add the volumes of both prisms
To find the total volume of the figure, sum the volumes of both prisms:
$$ V_{\text{total}} = V_1 + V_2 $$
Thus,
$$ V_{\text{total}} = 750 , \text{cubic feet} + 750 , \text{cubic feet} $$
Calculate:
$$ V_{\text{total}} = 1500 , \text{cubic feet} $$
The final volume of the figure is $1500$ cubic feet.
More Information
The volume calculation involved adding the volumes of two rectangular prisms. Each prism had specific dimensions, and using the formula for the volume of a rectangular prism allowed for straightforward calculations.
Tips
- Forgetting to separate the object into distinct prisms or miscalculating the dimensions of each prism.
- Not adding the volumes of both prisms correctly.
- Failing to use the correct volume formula for rectangular prisms.
AI-generated content may contain errors. Please verify critical information