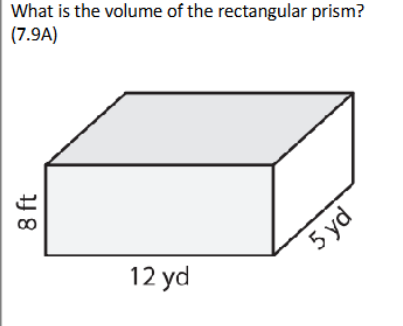
What is the volume of the rectangular prism?
Understand the Problem
The question asks us to calculate the volume of a rectangular prism given its dimensions. Note that the dimensions are given in mixed units, feet and yards, so a conversion will be needed to put them in the same units. A yard is three feet, so multiply the yards by 3 to convert to feet. Then the volume can be calculated by multiplying length, width, and height.
Answer
$4320 \text{ ft}^3$
Answer for screen readers
$4320 \text{ ft}^3$
Steps to Solve
- Convert yards to feet
Since 1 yard = 3 feet, we convert the dimensions in yards to feet.
$12 \text{ yd} = 12 \times 3 \text{ ft} = 36 \text{ ft}$ $5 \text{ yd} = 5 \times 3 \text{ ft} = 15 \text{ ft}$
- Calculate the volume
The volume $V$ of a rectangular prism is given by the product of its length $l$, width $w$, and height $h$. $V = lwh$
- Substitute values and compute
Substitute the given values into the volume formula $V = (8 \text{ ft}) \times (36 \text{ ft}) \times (15 \text{ ft})$ $V = 4320 \text{ ft}^3$
$4320 \text{ ft}^3$
More Information
The volume is expressed in cubic feet, $\text{ft}^3$, because we are multiplying three lengths together, each measured in feet.
Tips
A common mistake is forgetting to convert yards to feet before calculating the volume. Another mistake is using the wrong formula to calculate volume.
AI-generated content may contain errors. Please verify critical information