What is the value of x?
Understand the Problem
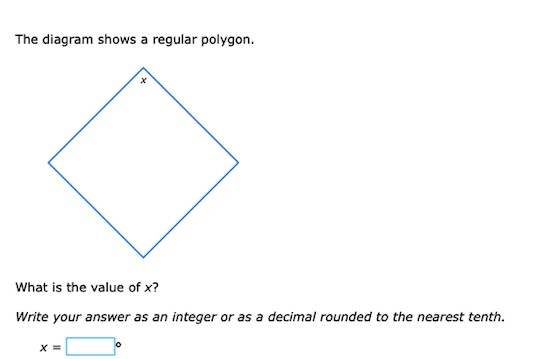
The question is asking for the value of x, which represents the measure of the interior angle of a regular polygon (specifically a square in this case, given the shape shown). To find x, we need to recall the formula for the interior angles of a polygon and apply it to this specific scenario.
Answer
$x = 90^\circ$
Answer for screen readers
$x = 90^\circ$
Steps to Solve
-
Identify the Polygon Type The figure is a square, which is a specific type of regular polygon with four equal sides and four equal angles.
-
Recall the Formula for Interior Angles The formula for calculating the measure of each interior angle of a regular polygon is given by: $$ \text{Interior Angle} = \frac{(n - 2) \cdot 180^\circ}{n} $$ where $n$ is the number of sides.
-
Substitute the Values For a square, $n = 4$. Applying this value into the formula: $$ \text{Interior Angle} = \frac{(4 - 2) \cdot 180^\circ}{4} $$
-
Calculate the Interior Angle Now calculate the value: $$ \text{Interior Angle} = \frac{2 \cdot 180^\circ}{4} = \frac{360^\circ}{4} = 90^\circ $$
-
Final Value of x Therefore, since $x$ represents one of the interior angles of a square, we conclude that: $$ x = 90^\circ $$
$x = 90^\circ$
More Information
In a square, all interior angles are equal and each measures $90^\circ$. This is a fundamental property of squares and rectangles.
Tips
- Confusing a square with other polygons; ensure you recognize the correct shape as a square.
- Misapplying the formula for a different type of polygon. Always check the number of sides.
AI-generated content may contain errors. Please verify critical information