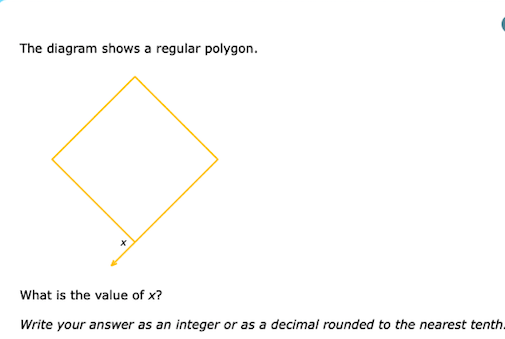
What is the value of x?
Understand the Problem
The question is asking to determine the value of x in the context of a regular polygon, which is likely referring to the internal angle or another property of the polygon.
Answer
The value of \( x \) is $90$.
Answer for screen readers
The value of ( x ) is $90$.
Steps to Solve
-
Identify the Polygon Type
The diagram shows a regular polygon that appears to be a square. In a square, all sides are equal, and all internal angles are equal to 90 degrees.
-
Determine the Internal Angles
For a square, the internal angles can be calculated as: [ \text{Internal Angle} = 90^\circ ]
-
Solve for ( x )
Given that ( x ) represents an internal angle of a square: [ x = 90^\circ ]
The value of ( x ) is $90$.
More Information
In a square, all angles are the same, and each measures $90$ degrees, which is a fundamental property of squares and rectangles.
Tips
- Confusing different types of polygons: Ensure you correctly identify that this is a square, as mistakes can occur by assuming shapes with similar visuals.
- Not recalling that all angles in a square are equal.
AI-generated content may contain errors. Please verify critical information