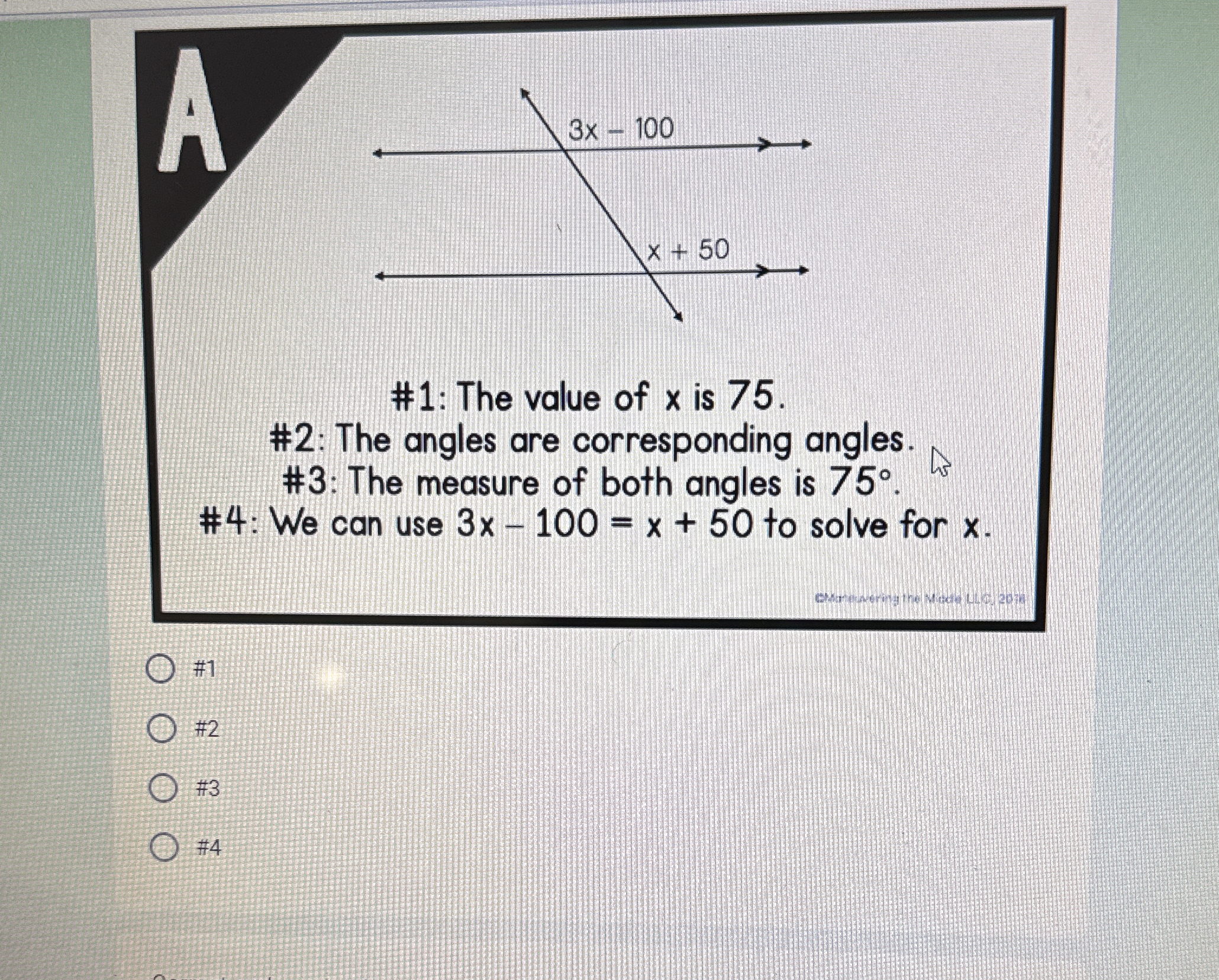
What is the value of x based on the equations given for the corresponding angles?

Understand the Problem

The question is asking to solve for the variable x based on given equations related to angles. The content suggests an understanding of corresponding angles and how to set up an equation to find x's value.

Answer

The value of $ x $ is $ 75 $.

Steps to Solve

Identify the relationship between angles

Since the angles are corresponding, they are equal. Thus, we can set up the equation:

$$ 3x - 100 = x + 50 $$

Rearranging the equation

To solve for $x$, first isolate $x$ by moving all terms involving $x$ to one side and constant terms to the other:

$$ 3x - x = 50 + 100 $$

Simplify the equation

Now simplify each side:

$$ 2x = 150 $$

Solve for x

Divide both sides by 2 to find the value of $x$:

$$ x = \frac{150}{2} $$

Final calculation

Calculate the value:

$$ x = 75 $$

The value of ( x ) is ( 75 ).

More Information

This problem involves corresponding angles, which are formed when a transversal crosses two parallel lines and create equal angles. The correct solution gives us the value of ( x ) directly related to those angles.

Tips

Forgetting to set the corresponding angles equal can lead to incorrect equations.
Misplacing signs when moving terms across the equal sign may result in calculation errors.
Failing to simplify correctly after rearranging the equation.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!