What is the value of x?

Understand the Problem

The question is asking for the value of x in a convex polygon, where the angles are expressed in terms of x. To solve it, the sum of the interior angles of the polygon needs to be used in conjunction with the provided angles to set up an equation.

Answer

The value of $ x $ is $ 58 $.

Steps to Solve

Determine the number of sides in the polygon

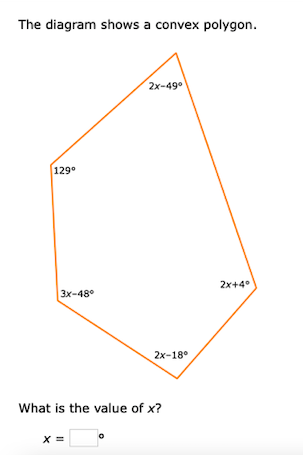
The polygon has 5 sides, as indicated by the 5 angles provided.

Calculate the sum of the interior angles

The formula for the sum of the interior angles of a polygon with ( n ) sides is:

$$ S = (n - 2) \times 180^\circ $$

Substituting ( n = 5 ):

$$ S = (5 - 2) \times 180^\circ = 3 \times 180^\circ = 540^\circ $$

Set up the equation based on the angles

The angles in the polygon are:

( 2x - 49^\circ )
( 3x - 48^\circ )
( 129^\circ )
( 2x + 4^\circ )
( 2x - 18^\circ )

Setting up the equation:

$$ (2x - 49) + (3x - 48) + 129 + (2x + 4) + (2x - 18) = 540 $$

Combine like terms

Combine all the ( x ) terms and the constant terms:

$$ 2x + 3x + 2x + 2x + 129 - 49 - 48 + 4 - 18 = 540 $$

This simplifies to:

$$ 9x + 18 = 540 $$

Solve for x

Subtract 18 from both sides:

$$ 9x = 540 - 18 $$

$$ 9x = 522 $$

Now divide by 9:

$$ x = \frac{522}{9} $$

Calculate the final value of x

Perform the division:

$$ x = 58 $$

The value of ( x ) is ( 58^\circ ).

More Information

The calculation of interior angles is crucial in geometry, particularly when determining properties of polygons. For a pentagon, the sum of the angles being ( 540^\circ ) is consistent across all convex polygons with five sides.

Tips

Some may forget to set the equation equal to ( 540^\circ ), leading to incorrect calculations.
Miscalculating the sum of the angles or misgrouping terms can cause errors. Always double-check combined terms.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!