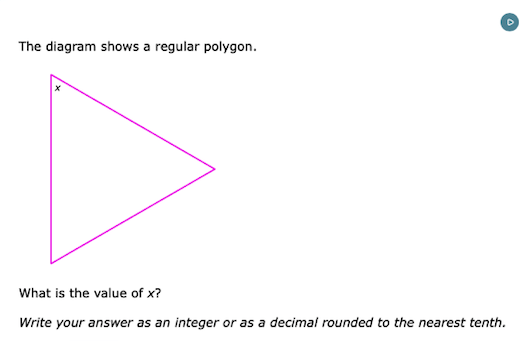
What is the value of x?
Understand the Problem
The question is asking for the value of the angle x in a regular polygon, which indicates we need to apply the knowledge of the properties of polygon angles to find the solution.
Answer
The value of \( x \) is \( 60 \).
Answer for screen readers
The value of ( x ) is ( 60 ).
Steps to Solve
- Identify the polygon type
From the diagram, we see that the polygon is a triangle, specifically an equilateral triangle because it is regular, meaning all sides and angles are equal.
- Calculate the angle of a regular triangle
In a regular triangle (equilateral triangle), each angle is the same. The formula for the interior angle of a regular polygon is:
$$ \text{Interior Angle} = \frac{(n-2) \times 180}{n} $$
For a triangle, ( n = 3 ):
$$ \text{Interior Angle} = \frac{(3-2) \times 180}{3} = \frac{180}{3} = 60 $$
- Determine the value of angle ( x )
Since the triangle is equilateral, each angle is ( 60^\circ ). Therefore, the value of ( x ) is:
$$ x = 60 $$
The value of ( x ) is ( 60 ).
More Information
In an equilateral triangle, all sides and angles are equal, making calculations straightforward. Each angle in such a triangle measures ( 60^\circ ).
Tips
- A common mistake is to incorrectly identify the type of polygon. Always verify the number of sides and angles.
- Confusing the interior angle formula for different types of polygons. Remember that regular polygons have specific properties.
AI-generated content may contain errors. Please verify critical information