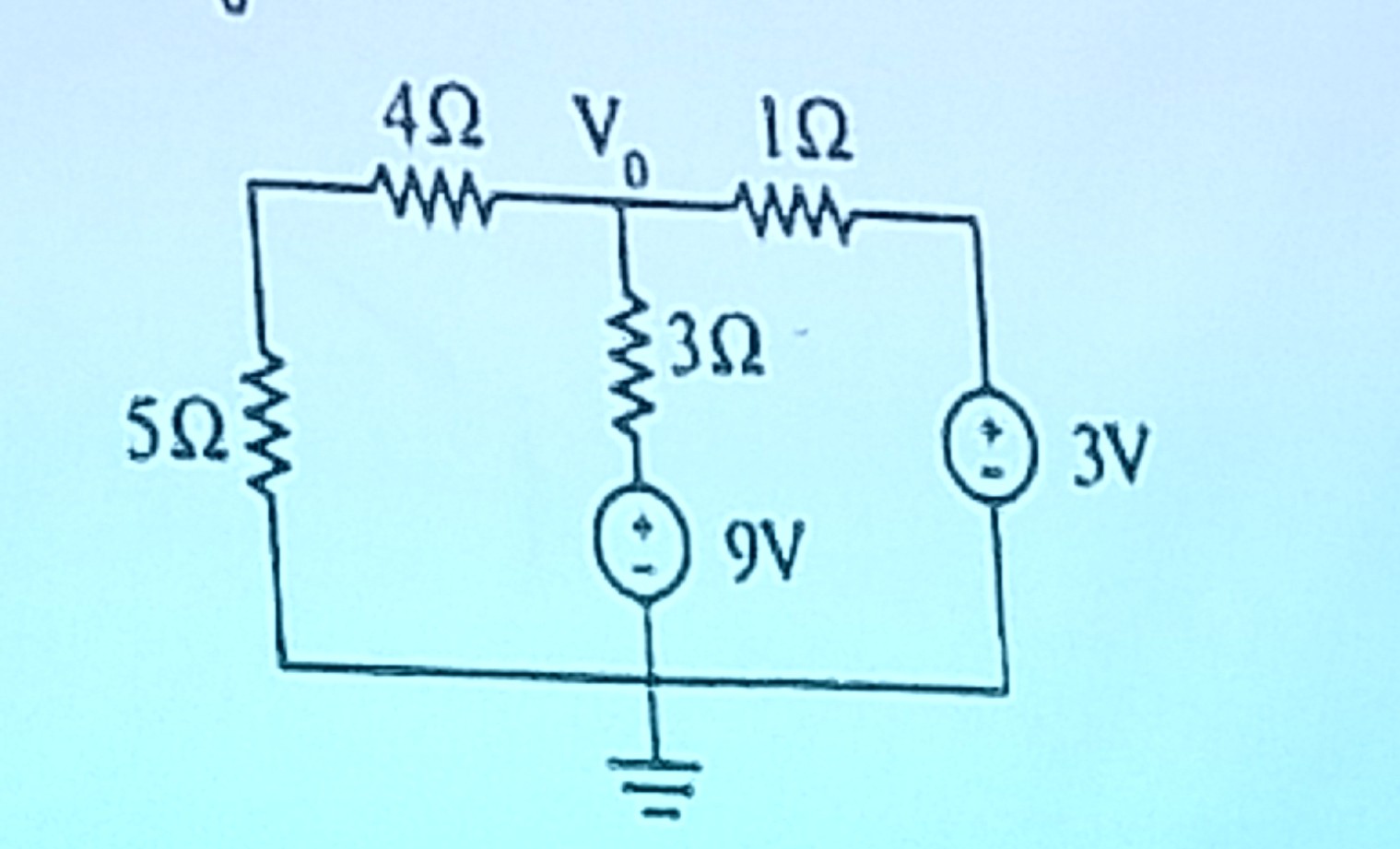
What is the value of V0 in the given circuit diagram?

Steps to Solve

1. Identify the components We have resistors of ( 4 , \Omega ), ( 1 , \Omega ), ( 3 , \Omega ), and ( 5 , \Omega ) as well as two voltage sources of ( 9V ) and ( 3V ).

2. Determine total resistance The ( 4 , \Omega ) and ( 1 , \Omega ) resistors are in series. Their equivalent resistance ( R_1 ) is calculated as: $$ R_1 = 4 , \Omega + 1 , \Omega = 5 , \Omega $$

3. Combine resistances in parallel Now the ( R_1 ) equivalent resistance (( 5 , \Omega )) is in parallel with the ( 3 , \Omega ) resistor. The total resistance ( R_{total} ) can be calculated using the formula for parallel resistors: $$ \frac{1}{R_{total}} = \frac{1}{R_1} + \frac{1}{3 , \Omega} $$ $$ \frac{1}{R_{total}} = \frac{1}{5} + \frac{1}{3} = \frac{3 + 5}{15} = \frac{8}{15} $$ Thus, $$ R_{total} = \frac{15}{8} , \Omega $$

4. Find the voltage in the circuit Next, apply the voltage sources. The effective voltage ( V_{source} ) in the loop is: $$ V_{source} = 9 , V - 3 , V = 6 , V $$

5. Calculate the total current Using Ohm’s Law, we can find the total current ( I ) flowing through the circuit: $$ I = \frac{V_{source}}{R_{total}} = \frac{6 , V}{\frac{15}{8} , \Omega} = \frac{6 \times 8}{15} = \frac{48}{15} = 3.2 , A $$

6. Determine voltage across resistors To find ( V_0 ) across the ( 1 , \Omega ) resistor, apply Ohm’s Law: $$ V_0 = I \cdot R = 3.2 , A \cdot 1 , \Omega = 3.2 , V $$