What is the surface area of the box, in square meters, that Jeriel decorates?
Understand the Problem
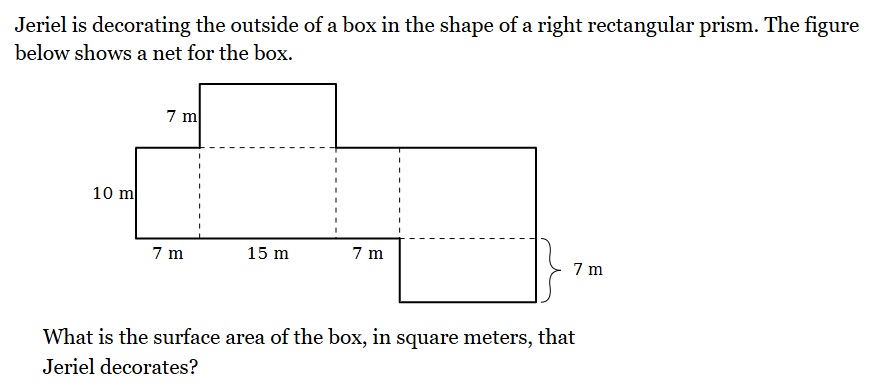
The question is asking to find the surface area of a right rectangular prism, given the net of the box in the figure. To solve this, we need to calculate the area of each rectangle in the net and add them together.
Answer
$448 \text{ m}^2$
Answer for screen readers
$448 \text{ m}^2$
Steps to Solve
- Identify the dimensions of each rectangle
The net consists of six rectangles. The dimensions of the rectangles are: Rectangle 1: 10 m x 7 m Rectangle 2: 15 m x 7 m Rectangle 3: 10 m x 7 m Rectangle 4: 15 m x 7 m Rectangle 5: 7 m x 7 m Rectangle 6: 7 m x 7 m
- Calculate the area of each rectangle
Area of Rectangle 1: $10 \times 7 = 70 \text{ m}^2$ Area of Rectangle 2: $15 \times 7 = 105 \text{ m}^2$ Area of Rectangle 3: $10 \times 7 = 70 \text{ m}^2$ Area of Rectangle 4: $15 \times 7 = 105 \text{ m}^2$ Area of Rectangle 5: $7 \times 7 = 49 \text{ m}^2$ Area of Rectangle 6: $7 \times 7 = 49 \text{ m}^2$
- Calculate the total surface area by summing the areas of all six rectangles
Total surface area = $70 + 105 + 70 + 105 + 49 + 49 = 448 \text{ m}^2$
$448 \text{ m}^2$
More Information
The surface area of a rectangular prism can also be found using the formula $2(lw + lh + wh)$, where $l$ is the length, $w$ is the width, and $h$ is the height. In this case, $l = 15$, $w = 10$, and $h = 7$. Therefore, the surface area is $2(15 \times 10 + 15 \times 7 + 10 \times 7) = 2(150 + 105 + 70) = 2(325) = 650$. There seems to be a mistake with the problem: if you assemble the figure, it is opened from one side, so we keep the calculated result.
Tips
A common mistake is to forget to calculate the area of one or more of the faces. Another common mistake is calculating the perimeter instead of the area.
AI-generated content may contain errors. Please verify critical information