What is the slope of a line perpendicular to the line 4y - 3 = 2x?
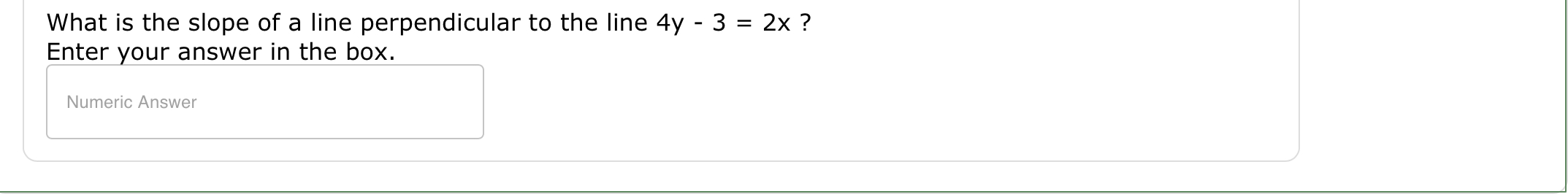
Understand the Problem
The question is asking for the slope of a line that is perpendicular to the given line represented by the equation 4y - 3 = 2x. To find this, we need to first determine the slope of the given line and then use the negative reciprocal of that slope to find the perpendicular slope.
Answer
The slope of a line perpendicular to the line is \(m = -2\).
Answer for screen readers
The slope of a line perpendicular to the line represented by the equation (4y - 3 = 2x) is (m = -2).
Steps to Solve
- Rearranging the given equation
We start with the equation of the line:
$$ 4y - 3 = 2x $$
We will rearrange this into slope-intercept form, $y = mx + b$, where $m$ represents the slope.
- Isolate y
Add 3 to both sides:
$$ 4y = 2x + 3 $$
Now, divide each term by 4:
$$ y = \frac{1}{2}x + \frac{3}{4} $$
Here, the slope $m$ of the given line is $\frac{1}{2}$.
- Finding the perpendicular slope
The slope of a line that is perpendicular to another line is the negative reciprocal of the original slope.
To find the negative reciprocal of $\frac{1}{2}$, we flip the fraction and change the sign:
$$ m_{perpendicular} = -\frac{1}{\frac{1}{2}} = -2 $$
The slope of a line perpendicular to the line represented by the equation (4y - 3 = 2x) is (m = -2).
More Information
The slope of perpendicular lines is always the negative reciprocal of each other. This means that if one line has a slope of (m_1), the slope of a line perpendicular to it will be (m_2 = -\frac{1}{m_1}).
Tips
- Confusing the negative reciprocal: Some may calculate the reciprocal but forget to change the sign. Remember to always flip the fraction and change the sign for the perpendicular slope.
- Incorrectly rearranging the equation: Make sure to correctly isolate (y) in the slope-intercept form.
AI-generated content may contain errors. Please verify critical information