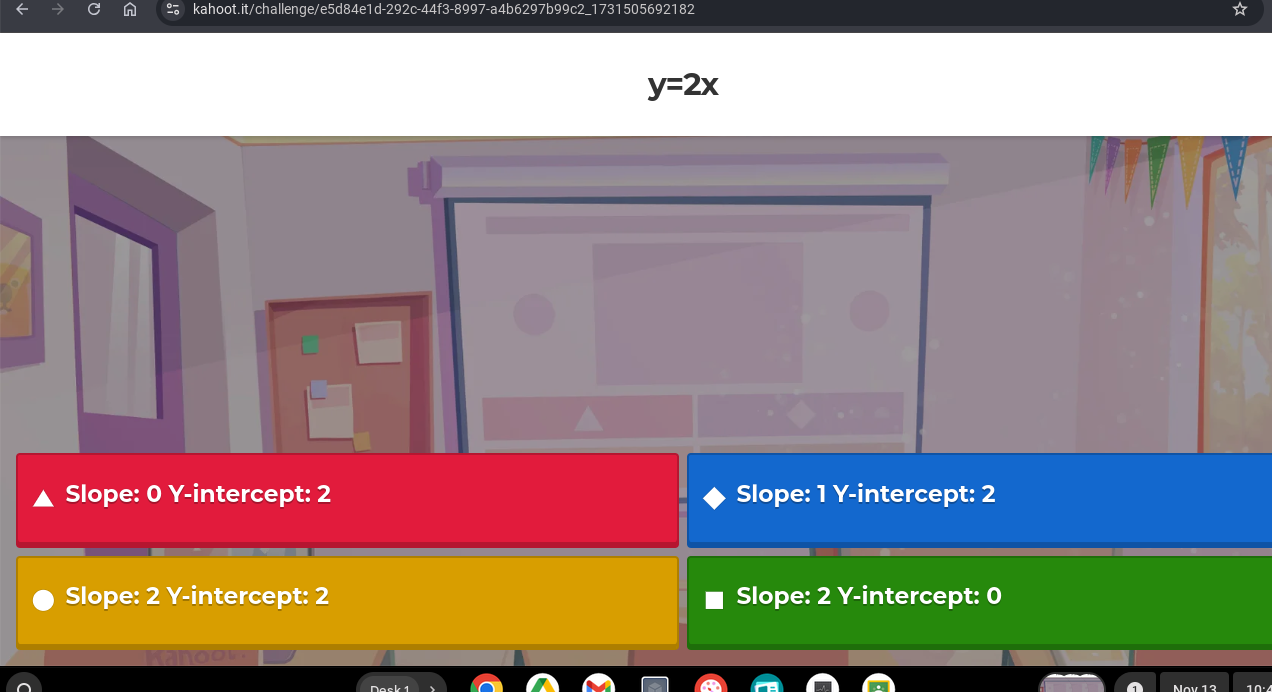
What is the slope and y-intercept of the equation y=2x?
Understand the Problem
The question is asking to identify the slope and y-intercept of the linear equation y=2x. In this case, the slope is the coefficient of x, and the y-intercept is the value of y when x equals 0.
Answer
Slope: $2$, Y-intercept: $0$
Answer for screen readers
The slope is $2$ and the y-intercept is $0$.
Steps to Solve
- Identify the slope and y-intercept in the equation
The given linear equation is $y = 2x$.
- Determine the slope
The slope ($m$) is the coefficient of $x$. In this case, $m = 2$.
- Determine the y-intercept
The y-intercept ($b$) is the value of $y$ when $x = 0$.
Substituting $x = 0$ into the equation: $$ y = 2(0) = 0 $$ So, the y-intercept is $b = 0$.
The slope is $2$ and the y-intercept is $0$.
More Information
In the equation $y = mx + b$, $m$ represents the slope, and $b$ represents the y-intercept. The slope indicates how steep the line is, while the y-intercept indicates where the line intersects the y-axis.
Tips
- Confusing the slope with the y-intercept. Remember, the slope is the coefficient of $x$, and the y-intercept is where the line crosses the y-axis when $x = 0$.
- Misreading the equation format. Ensure you identify the slope and intercept correctly from the standard linear equation form.
AI-generated content may contain errors. Please verify critical information