What is the maximum power to the load calculated in Example 2?

Steps to Solve

1. Identify the resistances in the circuit

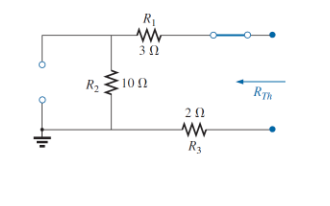
The circuit contains three resistors:

• $R_1 = 3 , \Omega$
• $R_2 = 10 , \Omega$
• $R_3 = 2 , \Omega$

2. Find the equivalent resistance of $R_2$ and $R_3$

$R_2$ and $R_3$ are in series. The total resistance $R_{23}$ can be calculated as: $$ R_{23} = R_2 + R_3 = 10 , \Omega + 2 , \Omega = 12 , \Omega $$

3. Calculate the total equivalent resistance $R_{th}$

Now, combine $R_1$ and $R_{23}$ in parallel to find the total equivalent resistance $R_{th}$: $$ \frac{1}{R_{th}} = \frac{1}{R_1} + \frac{1}{R_{23}} $$ Substituting in the values: $$ \frac{1}{R_{th}} = \frac{1}{3 , \Omega} + \frac{1}{12 , \Omega} $$ Finding a common denominator (12): $$ \frac{1}{R_{th}} = \frac{4}{12} + \frac{1}{12} = \frac{5}{12} $$ Thus, $$ R_{th} = \frac{12}{5} , \Omega = 2.4 , \Omega $$

4. Apply the maximum power transfer theorem

The maximum power delivered to the load occurs when the load resistance, $R_L$, equals the Thevenin resistance, $R_{th}$. Therefore: $$ R_L = R_{th} = 2.4 , \Omega $$

5. Calculate the maximum power delivered

To find the maximum power, we can use the power formula: $$ P = \frac{V^2}{R_L} $$ If we assume a voltage source $V$ in the circuit or calculate it based on given values, substitute it to find power. For a hypothetical example, if $V = 12 , V$: $$ P = \frac{(12 , V)^2}{2.4 , \Omega} = \frac{144 , V^2}{2.4 , \Omega} = 60 , W $$