What is 1 5/6 ÷ 2 1/5?
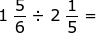
Understand the Problem
The question requires solving a division problem involving two mixed fractions. First, converting mixed fractions into improper fractions, then performing the division by multiplying by the reciprocal of the second fraction.
Answer
$\frac{5}{6}$
Answer for screen readers
$\frac{5}{6}$
Steps to Solve
- Convert the mixed fractions to improper fractions
Convert $1\frac{5}{6}$ to an improper fraction: $1\frac{5}{6} = \frac{(1 \times 6) + 5}{6} = \frac{6+5}{6} = \frac{11}{6}$
Convert $2\frac{1}{5}$ to an improper fraction: $2\frac{1}{5} = \frac{(2 \times 5) + 1}{5} = \frac{10+1}{5} = \frac{11}{5}$
- Rewrite the division problem with improper fractions
The original problem is $1\frac{5}{6} \div 2\frac{1}{5}$. Now, with improper fractions, the problem becomes: $\frac{11}{6} \div \frac{11}{5}$
- Divide the fractions by multiplying by the reciprocal
To divide by a fraction, multiply by its reciprocal. The reciprocal of $\frac{11}{5}$ is $\frac{5}{11}$. Thus, the problem becomes: $\frac{11}{6} \times \frac{5}{11}$
- Multiply the fractions
Multiply the numerators and the denominators: $\frac{11 \times 5}{6 \times 11} = \frac{55}{66}$
- Simplify the fraction
Simplify $\frac{55}{66}$ by dividing both the numerator and the denominator by their greatest common divisor, which is 11: $\frac{55 \div 11}{66 \div 11} = \frac{5}{6}$
$\frac{5}{6}$
More Information
The result of dividing $1\frac{5}{6}$ by $2\frac{1}{5}$ simplifies to $\frac{5}{6}$.
Tips
A common mistake is forgetting to convert mixed fractions into improper fractions before performing the division. Another mistake is forgetting to multiply by the reciprocal of the second fraction when dividing. Simplifying the fraction at the end is also often overlooked.
AI-generated content may contain errors. Please verify critical information