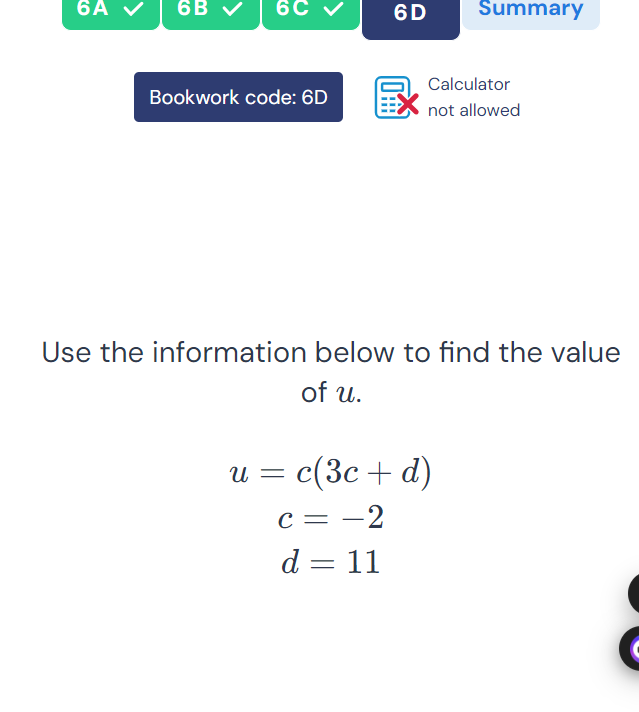
Use the information below to find the value of u: u = c(3c + d), c = -2, d = 11.
Understand the Problem
The question is asking us to calculate the value of 'u' using the provided equation and values for 'c' and 'd'. We will substitute the values of 'c' and 'd' into the equation and solve for 'u'.
Answer
The value of $u$ is $-10$.
Answer for screen readers
The final value of $u$ is
$$ u = -10 $$
Steps to Solve
- Substituting the values into the equation
Start by substituting the known values of $c$ and $d$ into the equation for $u$.
$$ u = c(3c + d) = (-2)(3(-2) + 11) $$
- Calculating the expression inside the parentheses
Calculate $3c + d$ using the substituted value of $c$ and $d$.
$$ 3(-2) + 11 = -6 + 11 = 5 $$
- Final multiplication to find u
Now substitute this result back into the equation to calculate $u$.
$$ u = -2 \times 5 = -10 $$
The final value of $u$ is
$$ u = -10 $$
More Information
The calculation shows how substituting variables and performing the operations step-by-step leads to the final value of $u$. Notice that even though $c$ is negative, it does not prevent obtaining a positive intermediate result in the parentheses.
Tips
- Misplacing negative signs: Ensure to keep track of negative signs when substituting values for $c$ and while calculating.
- Forgetting to apply operations in the correct order: Always follow the order of operations, especially when simplifying expressions inside parentheses.
AI-generated content may contain errors. Please verify critical information