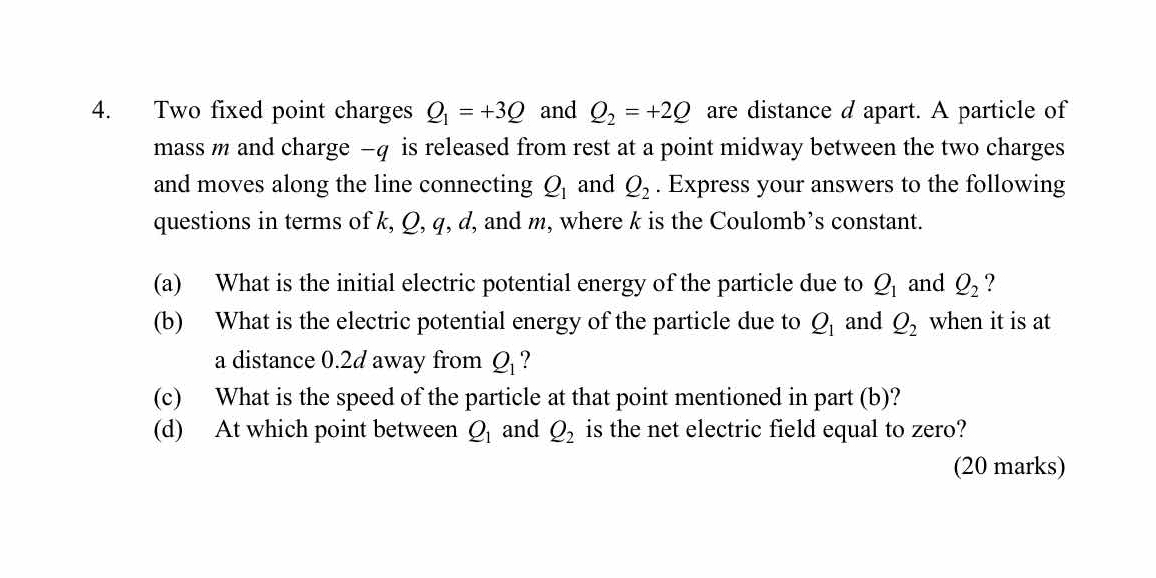
Two fixed point charges $Q_1 = +3Q$ and $Q_2 = +2Q$ are distance d apart. A particle of mass m and charge -q is released from rest at a point midway between the two charges and mov... Two fixed point charges $Q_1 = +3Q$ and $Q_2 = +2Q$ are distance d apart. A particle of mass m and charge -q is released from rest at a point midway between the two charges and moves along the line connecting $Q_1$ and $Q_2$. Express your answers to the following questions in terms of k, Q, q, d, and m, where k is the Coulomb's constant. (a) What is the initial electric potential energy of the particle due to $Q_1$ and $Q_2$? (b) What is the electric potential energy of the particle due to $Q_1$ and $Q_2$ when it is at a distance 0.2d away from $Q_1$? (c) What is the speed of the particle at that point mentioned in part (b)? (d) At which point between $Q_1$ and $Q_2$ is the net electric field equal to zero? Read more

Steps to Solve

1. (a) Initial Electric Potential Energy

The electric potential energy $U$ of a charge $-q$ due to another charge $Q$ at a distance $r$ is given by $U = k \frac{Qq}{r}$. Since the particle is midway between $Q_1$ and $Q_2$, its initial distance from each charge is $d/2$. The total initial electric potential energy $U_i$ is the sum of the potential energies due to $Q_1$ and $Q_2$: $$ U_i = k \frac{(3Q)(-q)}{d/2} + k \frac{(2Q)(-q)}{d/2} $$ $$ U_i = -\frac{2kq}{d}(3Q + 2Q) = -\frac{10kQq}{d} $$

2. (b) Electric Potential Energy at 0.2d from $Q_1$

When the particle is a distance $0.2d$ from $Q_1$, its distance from $Q_2$ is $d - 0.2d = 0.8d$. The electric potential energy $U_f$ at this point is: $$ U_f = k \frac{(3Q)(-q)}{0.2d} + k \frac{(2Q)(-q)}{0.8d} $$ $$ U_f = -\frac{kQq}{d} \left( \frac{3}{0.2} + \frac{2}{0.8} \right) = -\frac{kQq}{d} \left( 15 + 2.5 \right) = -\frac{17.5kQq}{d} $$

3. (c) Speed at 0.2d from $Q_1$

Use conservation of energy: the initial potential energy plus the initial kinetic energy equals the final potential energy plus the final kinetic energy. The initial kinetic energy is zero since the particle starts from rest. Therefore: $$ U_i + 0 = U_f + \frac{1}{2}mv^2 $$ $$ \frac{1}{2}mv^2 = U_i - U_f $$ $$ \frac{1}{2}mv^2 = -\frac{10kQq}{d} - \left( -\frac{17.5kQq}{d} \right) = \frac{7.5kQq}{d} $$ $$ v^2 = \frac{15kQq}{md} $$ $$ v = \sqrt{\frac{15kQq}{md}} $$

4. (d) Point of Zero Net Electric Field

The electric field due to a point charge $Q$ is $E = k \frac{Q}{r^2}$. Let $x$ be the distance from $Q_1$ where the net electric field is zero. Then the distance from $Q_2$ is $d - x$. We want the magnitudes of the electric fields to be equal: $$ k \frac{3Q}{x^2} = k \frac{2Q}{(d-x)^2} $$ $$ \frac{3}{x^2} = \frac{2}{(d-x)^2} $$ $$ 3(d-x)^2 = 2x^2 $$ $$ 3(d^2 - 2dx + x^2) = 2x^2 $$ $$ 3d^2 - 6dx + 3x^2 = 2x^2 $$ $$ x^2 - 6dx + 3d^2 = 0 $$ Use the quadratic formula to solve for $x$: $$ x = \frac{-(-6d) \pm \sqrt{(-6d)^2 - 4(1)(3d^2)}}{2(1)} $$ $$ x = \frac{6d \pm \sqrt{36d^2 - 12d^2}}{2} = \frac{6d \pm \sqrt{24d^2}}{2} = \frac{6d \pm 2d\sqrt{6}}{2} $$ $$ x = 3d \pm d\sqrt{6} $$ Since the point must be between $Q_1$ and $Q_2$, we must have $0 < x < d$. $3d + d\sqrt{6}$ is clearly greater than $d$, so the only valid solution is: $$ x = 3d - d\sqrt{6} = (3 - \sqrt{6})d $$