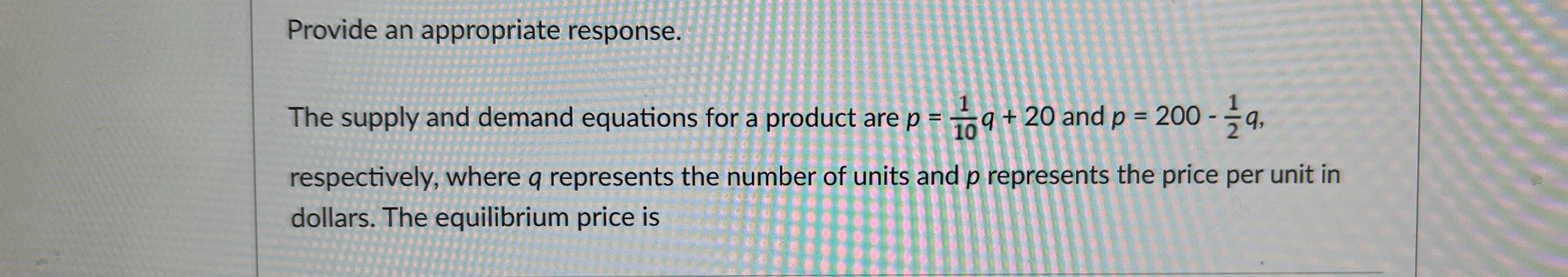
The supply and demand equations for a product are p = (1/10)q + 20 and p = 200 - (1/2)q, respectively, where q represents the number of units and p represents the price per unit in... The supply and demand equations for a product are p = (1/10)q + 20 and p = 200 - (1/2)q, respectively, where q represents the number of units and p represents the price per unit in dollars. The equilibrium price is? Read more

Steps to Solve

1. Set the supply and demand equations equal to each other Since we are looking for the equilibrium point, we need to find the point where supply equals demand. This means we set the two equations equal. $$ \frac{1}{10}q + 20 = 200 - \frac{1}{2}q $$

2. Solve for q Isolate $q$ by combining like terms. First add $\frac{1}{2}q$ to both sides of the equation $$ \frac{1}{10}q + \frac{1}{2}q + 20 = 200 $$ Rewrite $\frac{1}{2}$ as $\frac{5}{10}$ to have a common denominator: $$ \frac{1}{10}q + \frac{5}{10}q + 20 = 200 $$ $$ \frac{6}{10}q + 20 = 200$$ Subtract 20 from both sides: $$ \frac{6}{10}q = 180 $$ Multiply both sides by $\frac{10}{6}$: $$ q = 180 \cdot \frac{10}{6} $$ $$ q = 30 \cdot 10 = 300 $$

3. Substitute q back into either equation to find p Substitute $q = 300$ into the supply equation: $$ p = \frac{1}{10}(300) + 20 $$ $$ p = 30 + 20 = 50 $$ We can check with the demand equation: $$ p = 200 - \frac{1}{2}(300) $$ $$ p = 200 - 150 = 50 $$