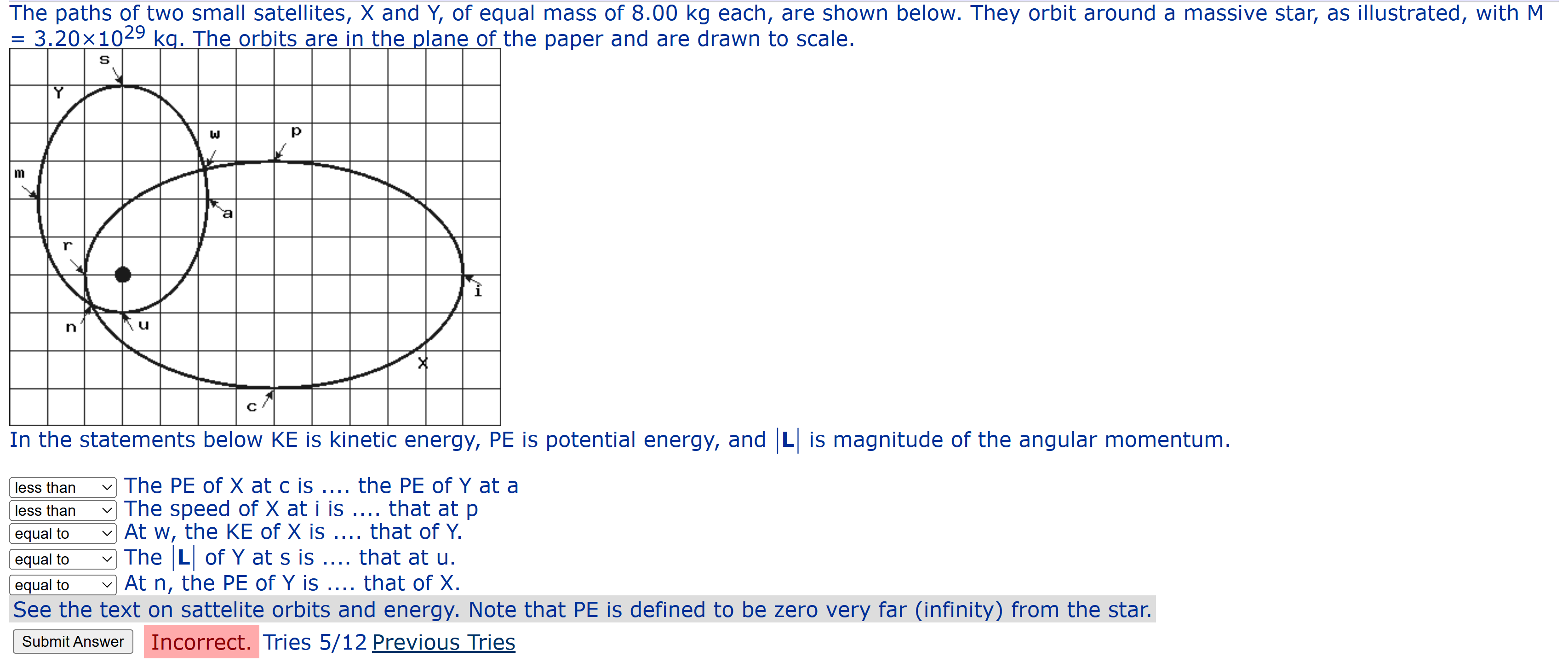
The PE of X at c is .... the PE of Y at a. The speed of X at i is .... that at p. At w, the KE of X is .... that of Y. The |L| of Y at s is .... that at u. At n, the PE of Y is ...... The PE of X at c is .... the PE of Y at a. The speed of X at i is .... that at p. At w, the KE of X is .... that of Y. The |L| of Y at s is .... that at u. At n, the PE of Y is .... that of X. Read more

Steps to Solve

1. Understanding Potential Energy (PE)

The potential energy (PE) between two masses due to gravity is given by the formula:

$$ PE = - \frac{GMm}{r} $$

Where:

• ( G ) = gravitational constant ( (6.674 \times 10^{-11} , m^3 , kg^{-1} , s^{-2}) )
• ( M ) = mass of the star ( (3.20 \times 10^{29} , kg) )
• ( m ) = mass of the satellite ( (8.00 , kg) )
• ( r ) = distance from the center of the star to the satellite

This implies that as ( r ) decreases (i.e., as the satellite gets closer to the star), ( PE ) becomes more negative.

2. Comparing PE at Points c and a

For satellite X at point c, the distance ( r ) is shorter compared to satellite Y at point a. Thus:

$$ PE_X(c) < PE_Y(a) $$

This means the PE of X at c is less than that of Y at a.

3. Understanding Kinetic Energy (KE)

The kinetic energy (KE) of an object in orbit can be described by the formula:

$$ KE = \frac{1}{2} mv^2 $$

Where ( v ) is the orbital speed. In a stable orbit, total mechanical energy is the sum of KE and PE.

4. Comparing Speed at Points i and p

At point i, satellite X is further from the star compared to satellite Y at point p. Therefore, satellite Y, which is closer, must move faster to maintain its orbit. Hence:

$$ v_X(i) < v_Y(p) $$

This means the speed of X at i is less than that of Y at p.

5. Comparing KE at Points w and a

Since Y is closer at point a, it has a higher speed than satellite X at w. Consequently, the kinetic energy will also be higher for Y:

$$ KE_X(w) < KE_Y(a) $$

Thus, the KE of X at w is less than that of Y at a.

6. Comparing Angular Momentum at Points s and u

The magnitude of angular momentum ( L ) is given as:

$$ L = mvr $$

Since satellite Y at s has a greater distance (radius) and is moving with a higher speed than satellite X at u, we determine:

$$ |L_Y(s)| > |L_X(u)| $$

This means the angular momentum of Y at s is greater than that at u.

7. Comparing PE at Points n and X

At point n, satellite Y is further from the star than satellite X. By the same reasoning with the potential energy formula, we find:

$$ PE_Y(n) > PE_X(X) $$

Hence, the PE of Y at n is greater than that of X.