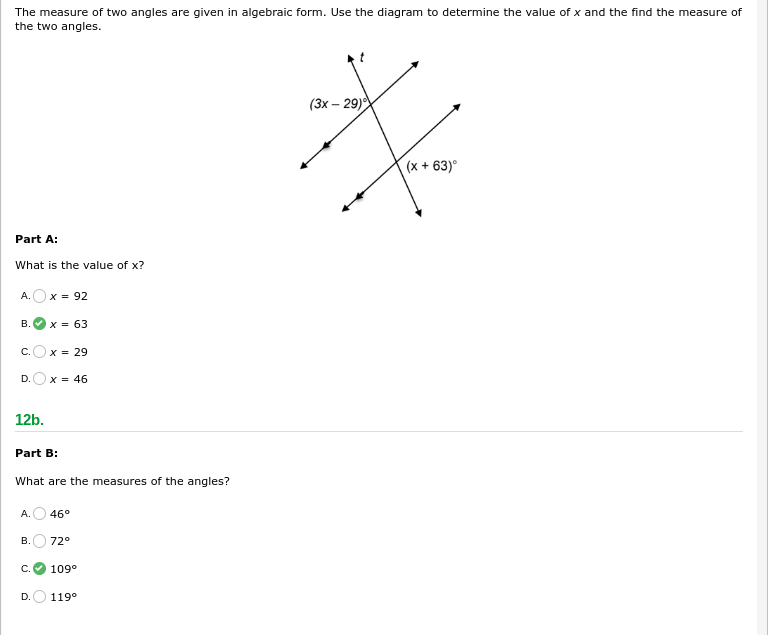
The measure of two angles are given in algebraic form. Use the diagram to determine the value of x and find the measure of the two angles.
Understand the Problem
The question is asking us to determine the value of x based on the algebraic expressions for the angles given in the diagram and also to find the measures of those angles.
Answer
The value of \( x \) is \( 46 \), and the measures of the angles are \( 109^\circ \).
Answer for screen readers
The value of ( x ) is ( 46 ). The measures of the angles are both ( 109^\circ ).
Steps to Solve
- Set up the equation using angle relationships
The angles ( (3x - 29)^\circ ) and ( (x + 63)^\circ ) are vertically opposite angles, so they are equal. Therefore, we can set up the equation: $$ 3x - 29 = x + 63 $$
- Simplify the equation
Now, we'll simplify the equation by isolating ( x ) on one side. First, subtract ( x ) from both sides: $$ 3x - x - 29 = 63 $$ This simplifies to: $$ 2x - 29 = 63 $$
- Solve for x
Next, add 29 to both sides to isolate ( 2x ): $$ 2x = 63 + 29 $$ This simplifies to: $$ 2x = 92 $$ Now, divide both sides by 2: $$ x = \frac{92}{2} $$ Thus, we find: $$ x = 46 $$
- Find the measures of the angles
Now that we know ( x ), we can find the measures of the angles: For the angle ( (3x - 29)^\circ ): $$ 3(46) - 29 = 138 - 29 = 109^\circ $$
For the angle ( (x + 63)^\circ ): $$ 46 + 63 = 109^\circ $$
The value of ( x ) is ( 46 ). The measures of the angles are both ( 109^\circ ).
More Information
The angles are equal because they are vertically opposite angles formed by two intersecting lines. This is a fundamental property in geometry.
Tips
- Misunderstanding angle relationships: Not recognizing that vertically opposite angles are equal.
- Algebraic errors: Incorrectly simplifying the equation can lead to an incorrect value for ( x .
AI-generated content may contain errors. Please verify critical information