The average of two numbers 'A' and 'B' is 20; that of 'B' and 'C' is 19; and that of 'C' and 'A' is 21. What is the value of 'A'?
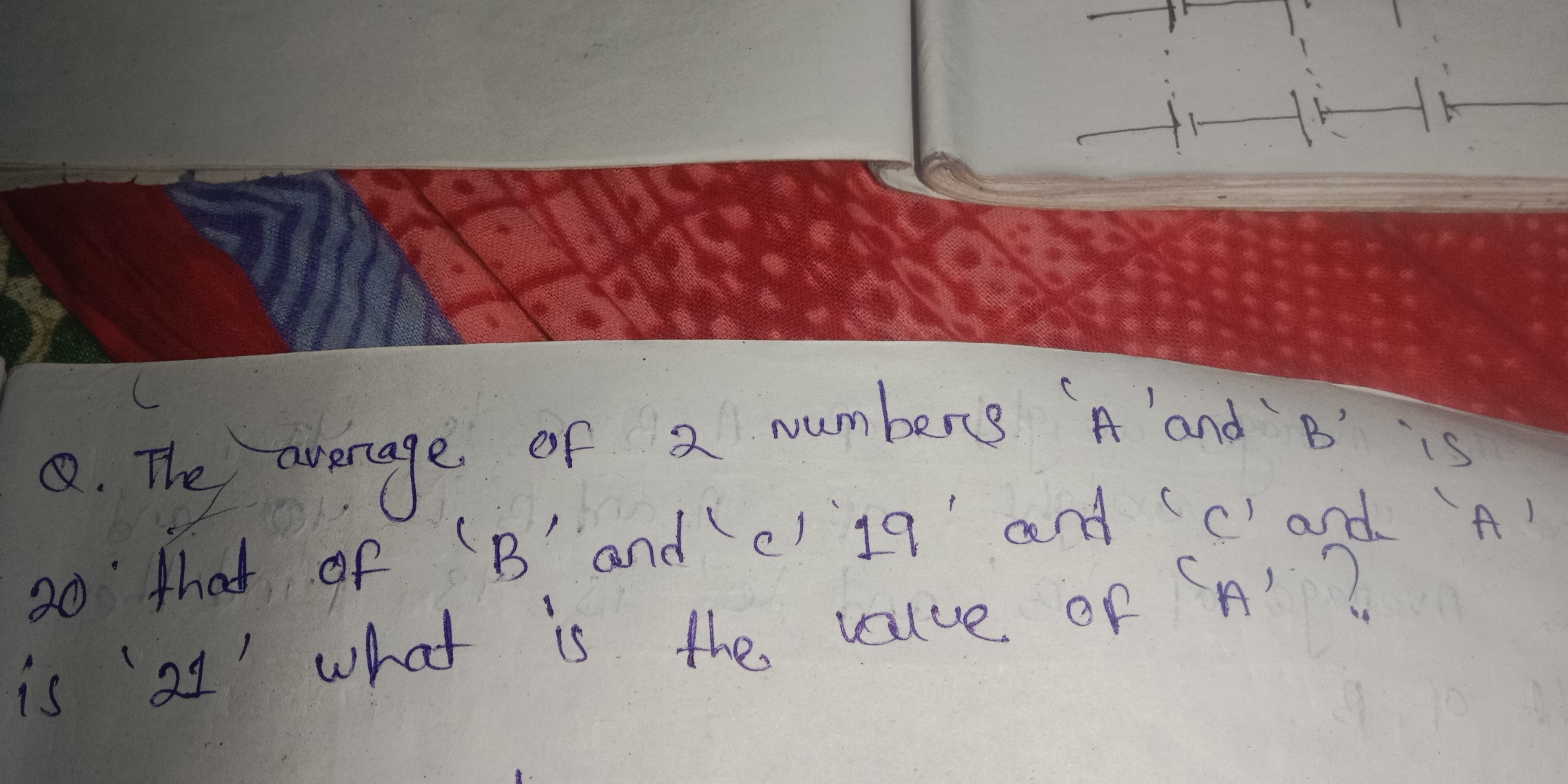
Understand the Problem
The question is asking for the value of 'A' based on the given averages of pairs of numbers: the average of 'A' and 'B' is 20, the average of 'B' and 'C' is 19, and the average of 'C' and 'A' is 21. We will need to set up equations based on these averages and solve for 'A'.
Answer
The value of \(A\) is \(22\).
Answer for screen readers
The value of (A) is (22).
Steps to Solve
- Set up equations from the averages
From the problem, we have the following averages:
-
The average of (A) and (B) is 20:
$$ \frac{A + B}{2} = 20 $$ Multiplying both sides by 2 gives:
$$ A + B = 40 , , , (1) $$
-
The average of (B) and (C) is 19:
$$ \frac{B + C}{2} = 19 $$ Multiplying both sides by 2 gives:
$$ B + C = 38 , , , (2) $$
-
The average of (C) and (A) is 21:
$$ \frac{C + A}{2} = 21 $$ Multiplying both sides by 2 gives:
$$ C + A = 42 , , , (3) $$
- Solve the equations
Now we have a system of equations:
- ( A + B = 40 )
- ( B + C = 38 )
- ( C + A = 42 )
We can solve these equations step by step.
- Express one variable in terms of another
From equation (1):
$$ B = 40 - A , , , (4) $$
Substituting equation (4) into equation (2):
$$ (40 - A) + C = 38 $$
Simplifying:
$$ C = 38 - 40 + A $$ $$ C = A - 2 , , , (5) $$
- Substituting C into equation (3)
Substituting equation (5) into equation (3):
$$ (A - 2) + A = 42 $$ This simplifies to:
$$ 2A - 2 = 42 $$
- Solve for A
Adding 2 to both sides gives:
$$ 2A = 44 $$
Dividing both sides by 2:
$$ A = 22 $$
The value of (A) is (22).
More Information
Finding the value of (A) involved setting up equations based on given averages and solving a system of equations. It showcases how relationships in averages can reveal unknown quantities.
Tips
- Ignoring the equations: Sometimes students forget to set up all required equations based on the averages. It's crucial to derive each equation carefully.
- Arithmetic errors: Mistakes in simple addition or subtraction can lead to incorrect final answers. Double-check your arithmetic.
AI-generated content may contain errors. Please verify critical information