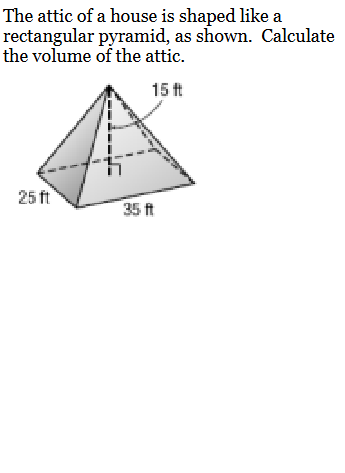
The attic of a house is shaped like a rectangular pyramid with dimensions 25 ft x 35 ft x 15 ft. Calculate the volume of the attic.
Understand the Problem
The question asks to calculate the volume of a rectangular pyramid, given the length, width, and height. We will use the formula for the volume of a rectangular pyramid, which is V = (1/3) * l * w * h, where l is the length, w is the width, and h is the height.
Answer
$4375 \text{ ft}^3$
Answer for screen readers
$4375 \text{ ft}^3$
Steps to Solve
- Identify the given values
From the problem, we have the following: Length $l = 35$ ft Width $w = 25$ ft Height $h = 15$ ft
- Apply the formula for the volume of a rectangular pyramid
The formula for the volume $V$ of a rectangular pyramid is: $V = \frac{1}{3} \cdot l \cdot w \cdot h$
- Substitute the given values into the formula and compute the volume
Substitute $l = 35$, $w = 25$, and $h = 15$ into the formula: $V = \frac{1}{3} \cdot 35 \cdot 25 \cdot 15$ $V = \frac{1}{3} \cdot 13125$ $V = 4375$
- State the final answer with correct units.
The volume of the attic is $4375$ cubic feet.
$4375 \text{ ft}^3$
More Information
The volume of the attic, which is shaped like a rectangular pyramid, is 4375 cubic feet. Volume is always measured in cubic units.
Tips
A common mistake is forgetting to multiply by $\frac{1}{3}$ when calculating the volume of a pyramid. Another mistake is using the wrong units, for example, $\text{ft}^2$ instead of $\text{ft}^3$ for volume.
AI-generated content may contain errors. Please verify critical information