Understand the Problem
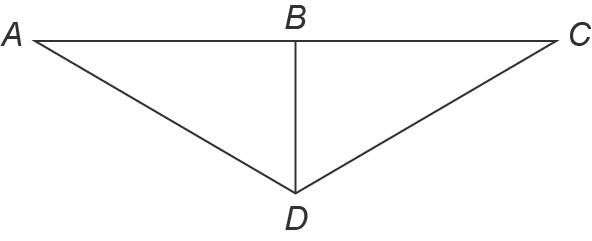
The question appears to involve a geometric figure with points A, B, C, and D. It likely asks for properties, calculations, or relationships between these points, such as angles or lengths in a triangle.
Answer
Key properties include angle relationships and potential congruence between triangles ABD and BDC.
Answer for screen readers
The specific answer will depend on additional information provided (e.g., side lengths, angles). However, the geometric properties show that triangles ABD and BDC are congruent due to the right angles and shared base.
Steps to Solve
-
Identify the Geometry The figure consists of a triangle formed by points A, D, and C with point B at the top. Notice how the point B is directly above the midpoint of line AC, forming two right triangles (ABD and BDC).
-
Determine Angles in the Triangles Since triangles ABD and BDC share the same base (line AC), we can infer angle relationships. Angle ADB and angle BDC are right angles as BD is perpendicular to AC.
-
Use Triangle Properties In triangles ABD and BDC, use the properties of right triangles:
- The sum of angles in each triangle equals $180^\circ$.
- If you know one angle, you can find the others.
- Calculate Lengths if Given (Hypothetical) If the lengths of AB or BC were provided, you could use the Pythagorean theorem to find lengths in the triangles: $$ AB^2 + AD^2 = BD^2 $$
The specific answer will depend on additional information provided (e.g., side lengths, angles). However, the geometric properties show that triangles ABD and BDC are congruent due to the right angles and shared base.
More Information
Triangles ABD and BDC can exhibit congruence, indicating properties such as equal angles and corresponding side lengths. This is fundamental in geometry, useful in proving aspects of the figure.
Tips
- Forgetting to account for right angles in calculations.
- Neglecting the properties of triangle congruence, leading to incorrect angle or length assumptions.
AI-generated content may contain errors. Please verify critical information