Solve using substitution. 8x - 3y = -7 -4x + 4y = 16

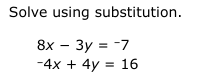
Understand the Problem

The question is asking to solve a system of equations using the substitution method. The equations given are linear and involve two variables, x and y.

Answer

The solution is $(1, 5)$.

Steps to Solve

Isolate one variable in one equation

Let's isolate $y$ in the first equation:

$$ 8x - 3y = -7 $$

Rearranging gives:

$$ -3y = -7 - 8x $$

Dividing by -3 yields:

$$ y = \frac{8x + 7}{3} $$

Substitute the expression for y into the other equation

Now, we substitute $y$ in the second equation:

$$ -4x + 4y = 16 $$

Substituting gives:

$$ -4x + 4\left(\frac{8x + 7}{3}\right) = 16 $$

Simplify and solve for x

Distributing $4$:

$$ -4x + \frac{32x + 28}{3} = 16 $$

Multiply through by 3 to eliminate the fraction:

$$ -12x + 32x + 28 = 48 $$

Combine like terms:

$$ 20x + 28 = 48 $$

Now, subtract 28:

$$ 20x = 20 $$

Dividing by 20:

$$ x = 1 $$

Substitute back to find y

Now substitute $x = 1$ back into the isolated equation for $y$:

$$ y = \frac{8(1) + 7}{3} = \frac{15}{3} = 5 $$

State the solution

The solution to the system of equations is:

$$ (x, y) = (1, 5) $$

The solution to the system of equations is $(1, 5)$.

More Information

The substitution method is a useful technique for solving systems of equations, especially when one equation can be easily manipulated to express one variable in terms of the other. The final answer indicates the values for $x$ and $y$ that satisfy both equations simultaneously.

Tips

Failing to correctly isolate one variable, leading to incorrect substitutions.
Miscalculating values during simplifications, especially when handling fractions.
Forgetting to substitute back into both equations to verify the solution.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!