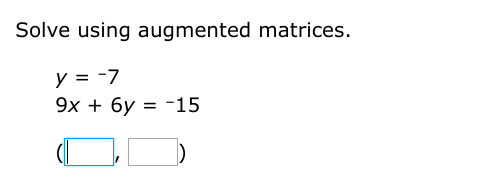
Solve using augmented matrices: y = -7, 9x + 6y = -15.
Understand the Problem
The question is asking to solve a system of equations using augmented matrices. It involves the equations y = -7 and 9x + 6y = -15, and we need to set up the corresponding augmented matrix and solve for the variables.
Answer
The solution is $x = 3, \, y = -7$.
Answer for screen readers
The solution to the system of equations is: $$x = 3, \quad y = -7$$
Steps to Solve
-
Set Up the Equations We have the two equations:
-
$y = -7$
-
$9x + 6y = -15$
-
Substitute y in the Second Equation Since we know $y = -7$, we can substitute this value into the second equation: $$9x + 6(-7) = -15$$
-
Simplify the Equation Let's simplify the equation: $$9x - 42 = -15$$
-
Isolate x Add 42 to both sides to isolate the term with $x$: $$9x = -15 + 42$$
-
Calculate the right side Calculate the simplification on the right: $$9x = 27$$
-
Solve for x Now, divide by 9 to solve for $x$: $$x = \frac{27}{9}$$ $$x = 3$$
-
Write the Augmented Matrix The corresponding augmented matrix for our system is: $$\begin{pmatrix} 0 & 1 & | & -7 \ 9 & 6 & | & -15 \end{pmatrix}$$
-
Final Values of x and y From our calculations: $$x = 3 \quad \text{and} \quad y = -7$$
The solution to the system of equations is: $$x = 3, \quad y = -7$$
More Information
This solution represents the values of the variables that satisfy both equations in the system. The method of using augmented matrices helps visualize and solve systems of equations in a structured way.
Tips
- Not substituting the value of $y$ correctly in the second equation.
- Forgetting to properly simplify each step or miscalculating the addition or subtraction.
- Making errors when dividing to isolate $x$.
AI-generated content may contain errors. Please verify critical information