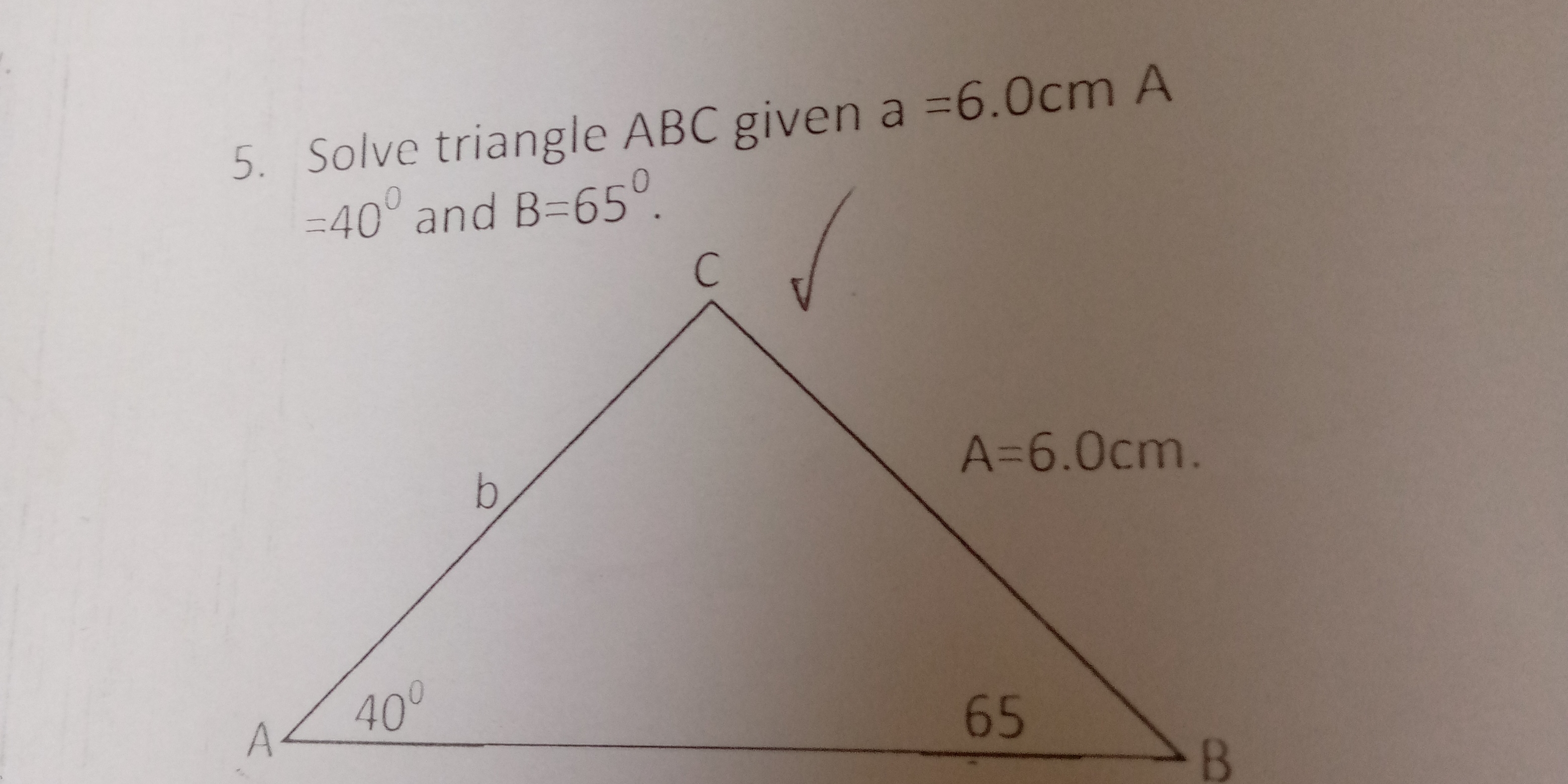
Solve triangle ABC given a = 6.0cm, A = 40°, and B = 65°.
Understand the Problem
The question asks to solve triangle ABC given side a = 6.0cm, angle A = 40 degrees, and angle B = 65 degrees. This involves finding the remaining angles and side lengths of the triangle using trigonometric principles such as the Law of Sines and the angle sum property of triangles.
Answer
$C = 75^\circ$, $b \approx 8.46 \text{ cm}$, $c \approx 9.03 \text{ cm}$
Answer for screen readers
$C = 75^\circ$ $b \approx 8.46 \text{ cm}$ $c \approx 9.03 \text{ cm}$
Steps to Solve
- Find angle C
The sum of angles in a triangle is 180 degrees. We have $A = 40^\circ$ and $B = 65^\circ$. Therefore, $$C = 180^\circ - A - B = 180^\circ - 40^\circ - 65^\circ = 75^\circ$$
- Find side b using the Law of Sines
The Law of Sines states that $\frac{a}{\sin A} = \frac{b}{\sin B} = \frac{c}{\sin C}$. We are given $a = 6.0$ cm and we know angles A, B, and C. We can find side b using: $$\frac{a}{\sin A} = \frac{b}{\sin B}$$ $$b = \frac{a \sin B}{\sin A} = \frac{6.0 \cdot \sin 65^\circ}{\sin 40^\circ}$$ $$b \approx \frac{6.0 \cdot 0.9063}{0.6428} \approx 8.46 \text{ cm}$$
- Find side c using the Law of Sines
Similarly, we can find side c using the Law of Sines: $$\frac{a}{\sin A} = \frac{c}{\sin C}$$ $$c = \frac{a \sin C}{\sin A} = \frac{6.0 \cdot \sin 75^\circ}{\sin 40^\circ}$$ $$c \approx \frac{6.0 \cdot 0.9659}{0.6428} \approx 9.03 \text{ cm}$$
$C = 75^\circ$ $b \approx 8.46 \text{ cm}$ $c \approx 9.03 \text{ cm}$
More Information
The Law of Sines is a powerful tool for solving triangles when you know an angle and its opposite side, along with another angle or side. Make sure your calculator is in degree mode when calculating trigonometric functions.
Tips
A common mistake is to forget to set your calculator to degree mode when computing the sine of angles expressed in degrees. Another frequent error is incorrect algebraic manipulation when solving for the unknown sides using the Law of Sines.
AI-generated content may contain errors. Please verify critical information