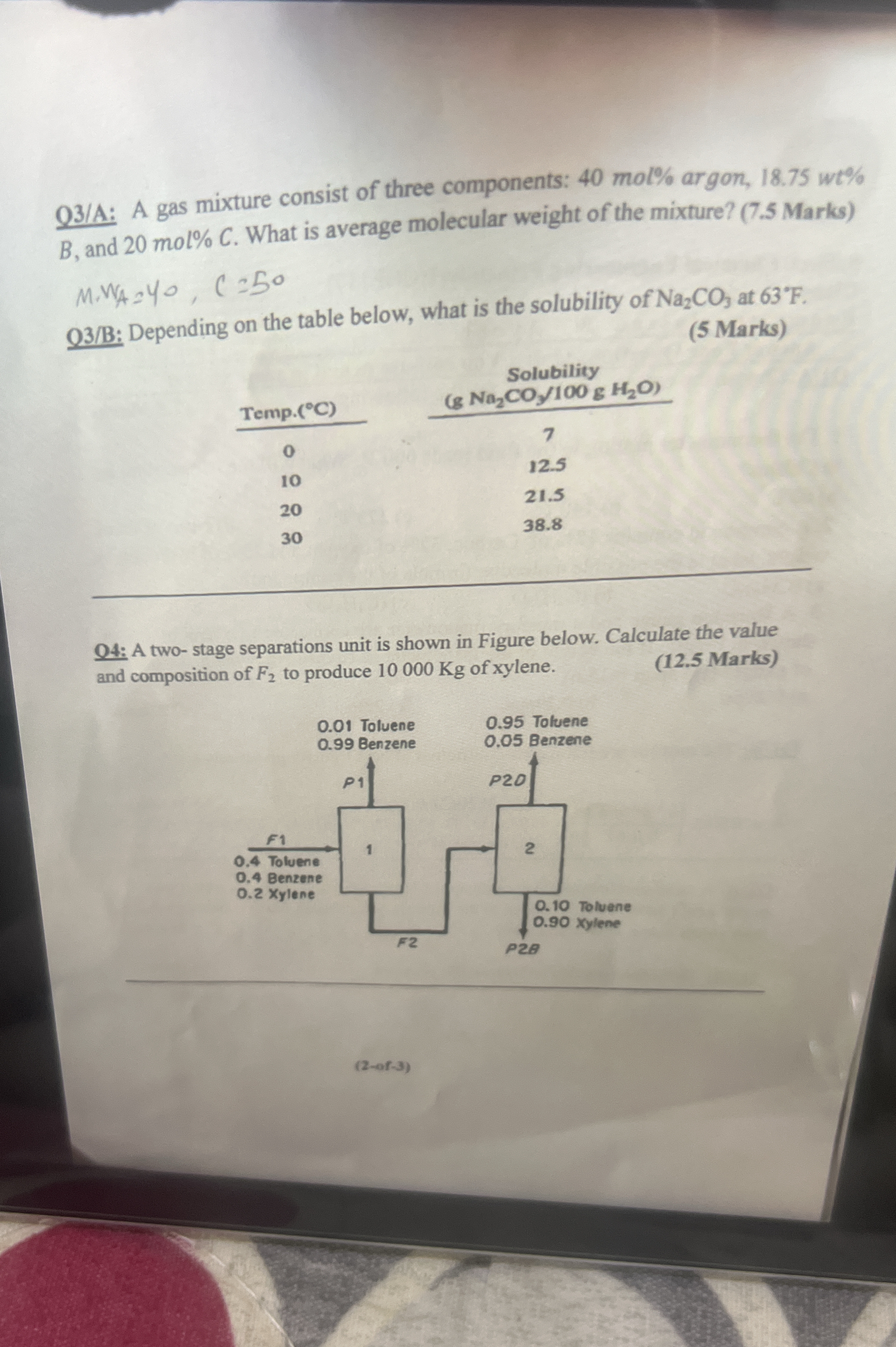
Solve these chemistry and chemical engineering questions: Q3/A: A gas mixture consists of three components: 40 mol% argon, 18.75 wt% B, and 20 mol% C. What is the average molecul... Solve these chemistry and chemical engineering questions: Q3/A: A gas mixture consists of three components: 40 mol% argon, 18.75 wt% B, and 20 mol% C. What is the average molecular weight of the mixture? Q3/B: Depending on the table below, what is the solubility of Na2CO3 at 63°F. Q4: A two-stage separations unit is shown in the figure. Calculate the value and composition of F2 to produce 10000 Kg of xylene. Read more

Steps to Solve

Here's a breakdown of how to solve each question:

Q3/A: Average Molecular Weight Calculation

1. Calculate the mole percentage of component B:

Since we know that the mixture consists of 40 mol% Argon and 20 mol% C, the remaining percentage must be component B. Therefore, the mole percentage of B ($mol%_B$) is calculated below: $mol%_B = 100% - 40% - 20% = 40%$

2. Assume a basis of 100 g of the mixture.:

If we assume the 100g basis, then the mass of B is 18.75 g. The mass fractions of the remaining components can be determined from the mole fractions in step 1.

3. Calculate the mass of Argon and C:

The mass of Argon ($m_{Ar}$) can be calculated from the mole fractions in step 1. We need to convert the moles of Argon to mass, then account for the fact that this mass represents the percentage not already accounted for by B (18.75wt%).

$Moles_{Ar} = 0.40 \ moles$

$m_{Ar} = 0.40 \ moles \times 40 \ g/mol = 16 \ g$

Similarly, the mass of C ($m_C$) can be calculated from the mole fractions $Moles_{C} = 0.20 \ moles$

$m_{C} = 0.20 \ moles \times 50 \ g/mol = 10 \ g$

4. Calculate the total mass for the 1 mole mixuture:

The total mass is $16 \ g + 18.75 \ g + 10 \ g = 44.75 \ g$

5. Calculate molecular weight of the mixture:

$MW_{mix} = 44.75 \ g/mol$

Q3/B: Solubility Determination

1. Convert Fahrenheit to Celsius:

Use the formula: $°C = \frac{5}{9}(°F - 32)$

$°C = \frac{5}{9}(63 - 32)$

$°C = \frac{5}{9}(31) \approx 17.22$

2. Interpolate the solubility:

The temperature 17.22°C lies between 10°C and 20°C. Interpolate between the solubility values at these temperatures (12.5 and 21.5 g $Na_2CO_3$/100 g $H_2O$ respectively).

$\newline$ $Solubility = 12.5 + \frac{(17.22 - 10)}{(20 - 10)} \times (21.5 - 12.5)$ $\newline$ $Solubility = 12.5 + \frac{7.22}{10} \times 9$ $\newline$ $Solubility = 12.5 + 0.722 \times 9$ $\newline$ $Solubility = 12.5 + 6.498 \approx 18.998$

Q4: Two-Stage Separation Unit Analysis

1. Overall Xylene Balance:

The process produces 10,000 kg of xylene. All of this xylene comes from F1. Therefore, the mass flow rate of xylene in F1 is equal to (0.2 * F1). This xylene ends up in P2B (0.90 * P2B which equals the 10,000 kg production rate). Using this, isolate for overall xylene mass balance:

$0.2F_1=0.9P_{2B}=10000 \ kg$

This yields the total mass of stream $F_1$ and $P_{2B}$.

$F_1 = \frac{10000}{0.2} = 50000 \ kg$

$P_{2B} = \frac{10000}{0.9} = 11111.11 \ kg$

2. Calculate Toluene Mass Flow Rate in $F_1$ and $P_{2B}$:

$Toluene_{F1} = 0.4 \times 50000 = 20000 \ kg$

$Toluene_{P2B} = 0.1 \times 11111.11 = 1111.11 \ kg$

3. Overall Toluene Balance

Toluene in $F_1$ must equal toluene in $P_{20}$ + toluene in $P_{2B}$.

$Toluene_{F1} = Toluene_{P20} + Toluene_{P2B}$

$20000 = Toluene_{P20} + 1111.11$

$Toluene_{P20} = 18888.89 \ kg$

4. Calculate Total Mass Flow Rate in $P_{20}$

We know the mass fraction and mass flow rate. From this, we solve for the value of $P_{20}$:

$0.95 \times P_{20} = 18888.89 \ kg$

$P_{20} = 19882.98 \ kg$

5. Flow Rate of stream 2: Stream 2 equals the sum of $P_{20}$ and $P_{2B}$: $Stream \ 2 = 19882.98 + 11111.11 = 30994.09 \ kg$

6. Overall Mass Balance for Benzene: Benzene in $F_1$ = Benzene in $P_1$ + Benzene in $P_{20}$

$Benzene_{F1} = 0.4 \times 50000 = 20000 \ kg$

$Benzene_{P20} = 0.05 \times 19882.98 = 994.15 \ kg$

Thus, $P_1 = \frac{Benzene_{F1} - Benzene_{P20}}{0.99} = \frac{20000 - 994.15}{0.99} = 19197.83 \ kg$

7. Calculate $F_2$ Value:

We can use the overall mass balance for the first separation unit to find $F_2$: $F_1 = P_1 + F_2$ $F_2 = 50000 - 19197.83 = 30802.17 \ kg$

8. Calculate $F_2$ Composition:

To find the composition, perform a mass balance for each component around the first separation unit.

Toluene Balance: $Toluene_{F1} = Toluene_{P1} + Toluene_{F2}$ $Toluene_{F2} = 20000 - (0.01 \times 19197.83) = 19808.02 \ kg$ Mass fraction of Toluene in $F_2 = \frac{19808.02}{30802.17} = 0.643$

Benzene Balance: $Benzene_{F1} = Benzene_{P1} + Benzene_{F2}$ $Benzene_{F2} = 20000 - (0.99 \times 19197.83) = 1005.85 \ kg$ Mass fraction of Benzene in $F_2 = \frac{1005.85}{30802.17} = 0.033$

Xylene Balance: $Xylene_{F1} = Xylene_{P1} + Xylene_{F2}$ Since there is no Xylene in P1 ($Xylene_{P1} = 0$), $Xylene_{F2} = Xylene_{F1} = 0.2 \times 50000 = 10000 \ kg$ Mass fraction of Xylene in $F_2 = \frac{10000}{30802.17} = 0.325$