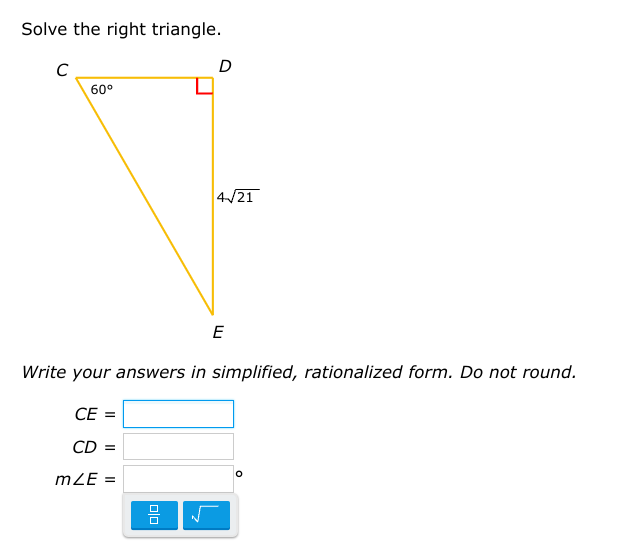
Solve the right triangle. Write your answers in simplified, rationalized form. Do not round. Find CE, CD, and m∠E.
Understand the Problem
The question asks to solve a right triangle given one angle and the length of one side. We need to determine the length of the other two sides (CE and CD) and the measure of angle E. We will use trigonometric ratios (sine, cosine, tangent) to find the missing side lengths and the fact that the sum of angles in a triangle is 180 degrees to find the missing angle.
Answer
$CE = 8\sqrt{7}$ $CD = 4\sqrt{7}$ $m\angle E = 30^\circ$
Answer for screen readers
$CE = 8\sqrt{7}$ $CD = 4\sqrt{7}$ $m\angle E = 30^\circ$
Steps to Solve
- Find angle $E$
Since the sum of angles in a triangle is $180^\circ$ and angle $D$ is a right angle ($90^\circ$), we have: $m\angle E = 180^\circ - 90^\circ - 60^\circ = 30^\circ$
- Find the length of $CE$
We know $DE = 4\sqrt{21}$ and $\angle C = 60^\circ$. We can use sine to find the length of the hypotenuse $CE$. $\sin(60^\circ) = \frac{DE}{CE}$ $CE = \frac{DE}{\sin(60^\circ)}$ $CE = \frac{4\sqrt{21}}{\frac{\sqrt{3}}{2}}$ $CE = \frac{4\sqrt{21} \cdot 2}{\sqrt{3}}$ $CE = \frac{8\sqrt{21}}{\sqrt{3}}$ $CE = \frac{8\sqrt{7 \cdot 3}}{\sqrt{3}}$ $CE = 8\sqrt{7}$
- Find the length of $CD$
We can use tangent to find the length of $CD$. $\tan(60^\circ) = \frac{DE}{CD}$ $CD = \frac{DE}{\tan(60^\circ)}$ $CD = \frac{4\sqrt{21}}{\sqrt{3}}$ $CD = \frac{4\sqrt{7 \cdot 3}}{\sqrt{3}}$ $CD = 4\sqrt{7}$
$CE = 8\sqrt{7}$ $CD = 4\sqrt{7}$ $m\angle E = 30^\circ$
More Information
The 30-60-90 triangle is a special right triangle. The sides are in the ratio $1:\sqrt{3}:2$.
Tips
A common mistake is to incorrectly identify the opposite and adjacent sides relative to the given angle or using the incorrect trigonometric ratio.
AI-generated content may contain errors. Please verify critical information