Solve the math problems in the image.
Understand the Problem
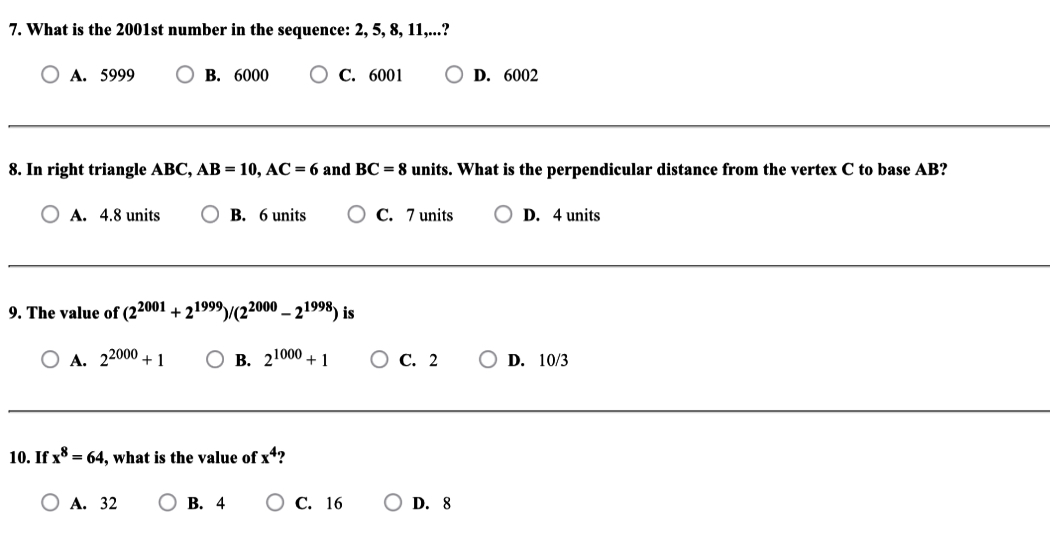
The provided image contains four math questions: 1) Finding the 2001st term in an arithmetic sequence. 2) Calculating the perpendicular distance from a vertex to the base of a right triangle. 3) Simplifying an expression with exponents. 4) Determining the value of (x^4) given (x^8 = 64).
Answer
7. 6002 8. 4.8 9. $\frac{10}{3}$ 10. 8
Answer for screen readers
- D. 6002
- A. 4.8 units
- D. 10/3
- D. 8
Steps to Solve
- Find the general term of the arithmetic sequence
The sequence is 2, 5, 8, 11,... The common difference $d$ is $5-2 = 3$. The first term $a_1$ is 2. The general term of an arithmetic sequence is given by $a_n = a_1 + (n-1)d$.
- Calculate the 2001st term
Substitute $n=2001$, $a_1 = 2$, and $d = 3$ into the formula for the nth term. $a_{2001} = 2 + (2001-1)(3) = 2 + (2000)(3) = 2 + 6000 = 6002$.
- Calculate the area of the right triangle using the legs
The legs of the right triangle are AC = 6 and BC = 8. The area of the triangle is given by $\frac{1}{2} \times base \times height = \frac{1}{2} \times 6 \times 8 = 24$ square units.
- Calculate the perpendicular distance from C to AB
Let the perpendicular distance from C to AB be $h$. The area of the triangle can also be calculated as $\frac{1}{2} \times AB \times h$. Since AB = 10, we have $\frac{1}{2} \times 10 \times h = 5h$. Equating the two area calculations, we have $5h = 24$, so $h = \frac{24}{5} = 4.8$ units.
- Simplify the expression with exponents
We have to simplify $\frac{2^{2001} + 2^{1999}}{2^{2000} - 2^{1998}}$. We can factor out common terms in the numerator and the denominator. Numerator: $2^{2001} + 2^{1999} = 2^{1999}(2^2 + 1) = 2^{1999}(4 + 1) = 5 \cdot 2^{1999}$ Denominator: $2^{2000} - 2^{1998} = 2^{1998}(2^2 - 1) = 2^{1998}(4 - 1) = 3 \cdot 2^{1998}$ Therefore, $\frac{2^{2001} + 2^{1999}}{2^{2000} - 2^{1998}} = \frac{5 \cdot 2^{1999}}{3 \cdot 2^{1998}} = \frac{5}{3} \cdot 2^{1999-1998} = \frac{5}{3} \cdot 2^1 = \frac{10}{3}$.
- Calculate $x^4$ given $x^8 = 64$
We are given $x^8 = 64$. We want to find $x^4$. Since $(x^4)^2 = x^8$, we can take the square root of both sides of the given equation: $\sqrt{x^8} = \sqrt{64}$ which gives $x^4 = 8$.
- D. 6002
- A. 4.8 units
- D. 10/3
- D. 8
More Information
Interesting to note for question 8 is that the side lengths of the triangle ABC form a Pythagorean triple.
Tips
-
For question 7, a common mistake is to forget to subtract 1 from the term number when using the formula for the nth term of an arithmetic sequence.
-
For question 8, a common mistake is to confuse which side is the base and which is the height, or to incorrectly calculate the area of the triangle.
-
For question 9, a common mistake is to incorrectly factor the terms in the numerator and the denominator, or to make a mistake when simplifying the exponents.
-
For question 10, a common mistake is to incorrectly take the square root of 64 or confuse the relationship between $x^4$ and $x^8$.
AI-generated content may contain errors. Please verify critical information