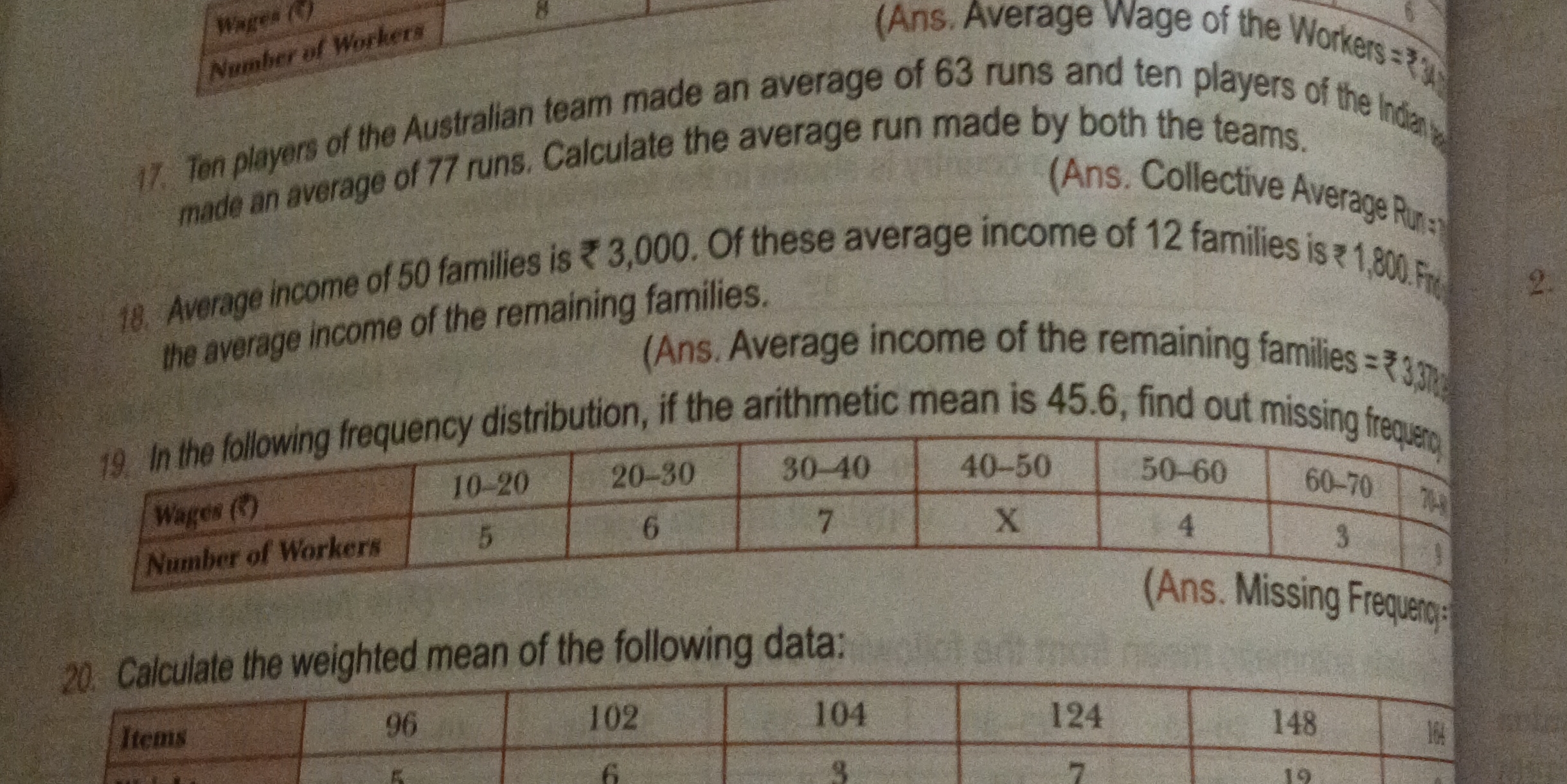
Solve the following problems: 1. Ten players of the Australian team made an average of 63 runs and ten players of the Indian team made an average of 77 runs. Calculate the average... Solve the following problems: 1. Ten players of the Australian team made an average of 63 runs and ten players of the Indian team made an average of 77 runs. Calculate the average run made by both the teams. 2. Average income of 50 families is ₹3,000. Of these, the average income of 12 families is ₹1,800. Find the average income of the remaining families. 3. In the following frequency distribution, if the arithmetic mean is 45.6, find out the missing frequency: Wages: 10-20, 20-30, 30-40, 40-50, 50-60, 60-70 Workers: 5, 6, 7, X, 4, 3
Understand the Problem
The image presents three math problems: 1) Calculate the average run made by two teams given their individual average runs and number of players. 2) Find the average income of the remaining families after some families' average income is known. 3) Determine the missing frequency in a frequency distribution given the arithmetic mean. These are standard statistics and average related questions.
Answer
$70$
Answer for screen readers
The average run made by both teams is $70$.
Steps to Solve
- Calculate the total runs for the Australian team
Multiply the number of players by their average runs: $10 \times 63 = 630$ runs.
- Calculate the total runs for the Indian team
Multiply the number of players by their average runs: $10 \times 77 = 770$ runs.
- Calculate the combined total runs
Add the total runs of both teams: $630 + 770 = 1400$ runs.
- Calculate the total number of players
Add the number of players from both teams: $10 + 10 = 20$ players.
- Calculate the average runs for both teams
Divide the combined total runs by the total number of players: $1400 / 20 = 70$ runs.
The average run made by both teams is $70$.
More Information
The average run rate of the combined teams is exactly halfway between the individual average run rates since each team included the same number of players (10).
Tips
A common mistake is to calculate the average of averages i.e. $(63 + 77)/2$. You can only average averages in such a way if both samples are the exact same size. In this case, it works fine (and gives $70$) because both teams have 10 players, but if one team had 9 players and the other had 10, then it would be incorrect to calculate this.
AI-generated content may contain errors. Please verify critical information