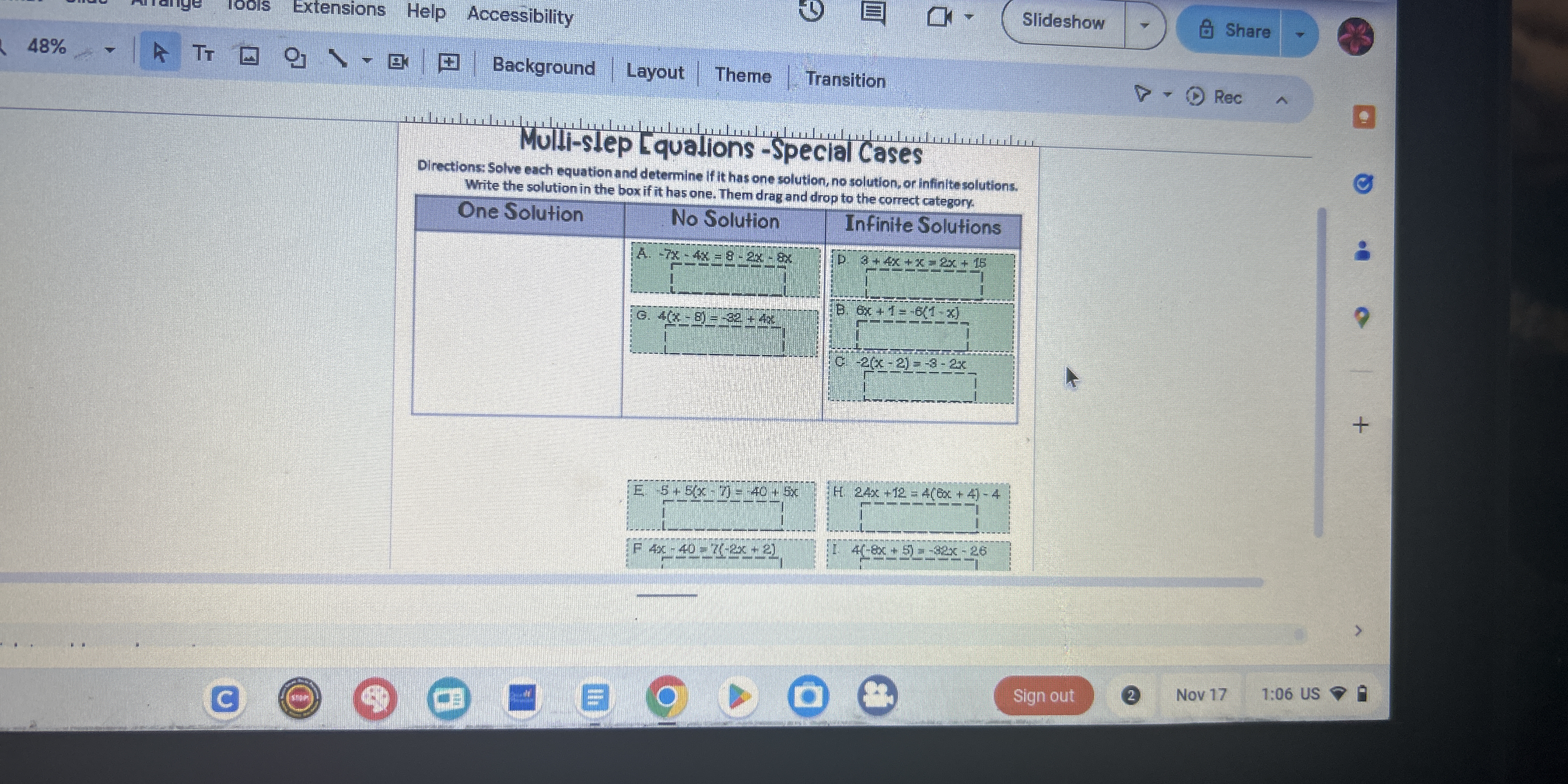
Solve the equations and determine if they have one solution, no solution, or infinite solutions.
Understand the Problem
The question is asking to solve a series of multi-step equations and categorize each one based on whether it has one solution, no solution, or infinite solutions.
Answer
A: $-\frac{4}{5}$, B: $\frac{17}{3}$, C: $\frac{7}{4}$, D: No solution, E: $\frac{21}{4}$, F: $\frac{23}{4}$, G: Infinite solutions, H: $\frac{3}{4}$, I: $\frac{43}{32}$.
Answer for screen readers
- A: $x = -\frac{4}{5}$
- B: $x = \frac{17}{3}$
- C: $x = \frac{7}{4}$
- D: No solution
- E: $x = \frac{21}{4}$
- F: $x = \frac{23}{4}$
- G: Infinite solutions
- H: $x = \frac{3}{4}$
- I: $x = \frac{43}{32}$
Steps to Solve
-
Equation A: Solve $-7x - 4 = 8x + 8$
Start by isolating the variable on one side. Move the $8x$ to the left side:
$$ -7x - 8x = 8 + 4 $$
This simplifies to:
$$ -15x = 12 $$
Divide both sides by -15:
$$ x = -\frac{12}{15} = -\frac{4}{5} $$
This has one solution: $x = -\frac{4}{5}$.
-
Equation B: Solve $x + 1 = 18 - 2x$
First, bring all $x$ terms to one side:
$$ x + 2x = 18 - 1 $$
This simplifies to:
$$ 3x = 17 $$
Divide by 3:
$$ x = \frac{17}{3} $$
This has one solution: $x = \frac{17}{3}$.
-
Equation C: Solve $2(x - 2) = 3 - 2x$
Distribute the left side:
$$ 2x - 4 = 3 - 2x $$
Add $2x$ to both sides:
$$ 4x - 4 = 3 $$
Then add 4:
$$ 4x = 7 $$
Divide by 4:
$$ x = \frac{7}{4} $$
This has one solution: $x = \frac{7}{4}$.
-
Equation D: Solve $2x - 4 = 2x + 15$
Subtract $2x$ from both sides:
$$ -4 = 15 $$
This is a contradiction, so it has no solution.
-
Equation E: Solve $5(5x + 7) = 140 + 5x$
Distribute:
$$ 25x + 35 = 140 + 5x $$
Subtract $5x$ from both sides:
$$ 20x + 35 = 140 $$
Now, subtract 35:
$$ 20x = 105 $$
Divide by 20:
$$ x = \frac{105}{20} = \frac{21}{4} $$
This has one solution: $x = \frac{21}{4}$.
-
Equation F: Solve $4x - 10 = 40 - 2(2x + 2)$
Start by simplifying the right side:
$$ 4x - 10 = 40 - 4x - 4 $$
Combine terms:
$$ 4x - 10 = 36 - 4x $$
Add $4x$ to both sides:
$$ 8x - 10 = 36 $$
Add 10:
$$ 8x = 46 $$
Divide by 8:
$$ x = \frac{46}{8} = \frac{23}{4} $$
This has one solution: $x = \frac{23}{4}$.
-
Equation G: Solve $4(x - B) = 32$
Divide both sides by 4:
$$ x - B = 8 $$
This simplifies to:
$$ x = 8 + B $$
This is variable dependent and has infinite solutions.
-
Equation H: Solve $2(4x + 12) = 4(6x + 4) - 4$
Distributing on both sides:
$$ 8x + 24 = 24x + 16 - 4 $$
Simplifying right side:
$$ 8x + 24 = 24x + 12 $$
Rearranging:
$$ 8x - 24x = 12 - 24 $$
This simplifies to:
$$ -16x = -12 $$
Hence,
$$ x = \frac{12}{16} = \frac{3}{4} $$
This has one solution: $x = \frac{3}{4}$.
-
Equation I: Solve $4(8x - 15) = -32x + 26$
Distribute on left side:
$$ 32x - 60 = -32x + 26 $$
Adding $32x$ to both sides:
$$ 64x - 60 = 26 $$
Add 60:
$$ 64x = 86 $$
Divide by 64:
$$ x = \frac{86}{64} = \frac{43}{32} $$
This has one solution: $x = \frac{43}{32}$.
- A: $x = -\frac{4}{5}$
- B: $x = \frac{17}{3}$
- C: $x = \frac{7}{4}$
- D: No solution
- E: $x = \frac{21}{4}$
- F: $x = \frac{23}{4}$
- G: Infinite solutions
- H: $x = \frac{3}{4}$
- I: $x = \frac{43}{32}$
More Information
Each equation is categorized based on whether it yields one solution, no solution, or infinitely many solutions. Equations leading to contradictions or identities have been identified accordingly.
Tips
- Misallocating terms when moving variables across the equal sign: Ensure sign changes are accurately applied.
- Failing to simplify before solving may lead to complex equations that are harder to manage.
- Misinterpreting infinite solutions: Check if the equation reduces to an identity (e.g., true for all values).
AI-generated content may contain errors. Please verify critical information