Solve the complex number problems.
Understand the Problem
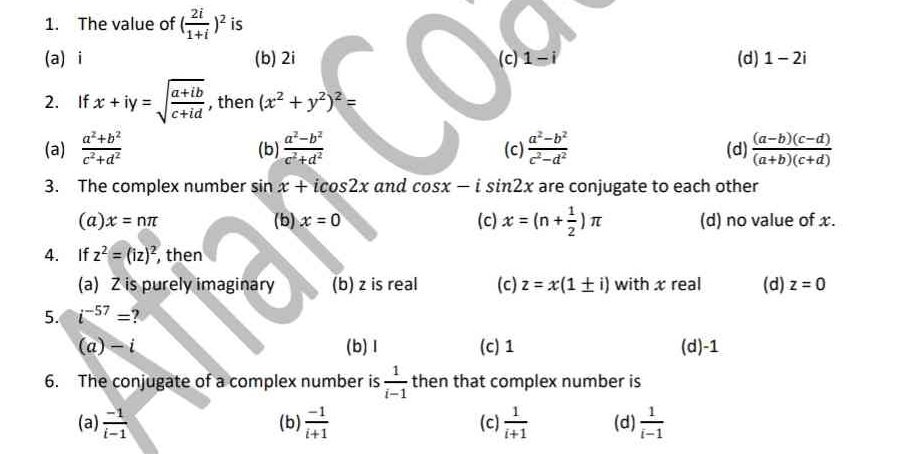
The image contains multiple choice questions related to complex numbers, which require applying various properties and operations to solve them. Here's a breakdown of each question:
- Calculate the value of a complex number expression raised to a power.
- Find the value of an expression involving squares, given that a complex number equals the square root of a fraction with complex numbers.
- Determine the possible values of x, given that two complex numbers are conjugates.
- Determine the value of z, given an equation involving the square of z and the square of iz.
- Compute the value of i raised to the power of -57.
- Find the complex number, given its conjugate.
We will classify these as math questions.
Answer
1. $2i$ 2. $\frac{a^2+b^2}{c^2+d^2}$ 3. no value of x. 4. z = 0 5. -i 6. $\frac{-1}{i+1}$
Answer for screen readers
- (b) $2i$
- (a) $\frac{a^2+b^2}{c^2+d^2}$
- (d) no value of x.
- (d) z = 0
- (a) -i
- (b) $\frac{-1}{i+1}$
Steps to Solve
- Calculate $(\frac{2i}{1+i})^2$
Simplify the expression inside the parenthesis first by multiplying the numerator and denominator by the conjugate of the denominator: $$ \frac{2i}{1+i} = \frac{2i(1-i)}{(1+i)(1-i)} = \frac{2i - 2i^2}{1 - i^2} = \frac{2i + 2}{1 + 1} = \frac{2 + 2i}{2} = 1 + i $$ Now, square the result: $$ (1+i)^2 = (1+i)(1+i) = 1 + 2i + i^2 = 1 + 2i - 1 = 2i $$
- Find $(x^2 + y^2)^2$ given $x + iy = \sqrt{\frac{a+ib}{c+id}}$
First, find the magnitude squared of $x+iy$: $$ |x+iy|^2 = x^2 + y^2 $$ Given that $x + iy = \sqrt{\frac{a+ib}{c+id}}$, then $$ (x+iy)^2 = \frac{a+ib}{c+id} $$ Then, $$ |(x+iy)^2| = |x+iy|^2 = |\frac{a+ib}{c+id}| = \frac{|a+ib|}{|c+id|} = \frac{\sqrt{a^2+b^2}}{\sqrt{c^2+d^2}} $$ So, $$ x^2 + y^2 = \sqrt{\frac{a^2+b^2}{c^2+d^2}} $$ Squaring both sides: $$ (x^2 + y^2)^2 = \frac{a^2 + b^2}{c^2 + d^2} $$
- Find x when $\sin x + i\cos 2x$ and $\cos x - i\sin 2x$ are conjugates
For two complex numbers to be conjugates, their real parts must be equal, and their imaginary parts must be the negative of each other. Therefore, $$ \sin x = \cos x $$ $$ \cos 2x = \sin 2x $$ From $\sin x = \cos x$, we have $x = (n + \frac{1}{4})2\pi = 2n\pi + \frac{\pi}{4}$ or $x = n\pi + \frac{\pi}{4} $, where $n$ is an integer. From $\cos 2x = \sin 2x$, $2x = n\pi + \frac{\pi}{4}$ or $x = n\frac{\pi}{2} + \frac{\pi}{8}$, where $n$ is an integer.
If $x = n\frac{\pi}{2} + \frac{\pi}{8}$, $\sin(n\frac{\pi}{2} + \frac{\pi}{8}) = \cos(n\frac{\pi}{2} + \frac{\pi}{8})$. This implies that $x = \frac{\pi}{4}$. If we substitute $x = \frac{\pi}{4}$, $\sin x = \sin \frac{\pi}{4} = \frac{\sqrt{2}}{2} = \frac{1}{\sqrt{2}}$ and $\cos x = \cos \frac{\pi}{4} = \frac{\sqrt{2}}{2} = \frac{1}{\sqrt{2}}$. Also, $\cos 2x = \cos \frac{\pi}{2} = 0 $ and $\sin 2x = \sin \frac{\pi}{2} = 1 $. Since $\cos 2x = \sin 2x$ is not possible, then there is no solution.
- If $z^2 = (iz)^2$, then find z
Given $z^2 = (iz)^2$, we have $z^2 = i^2 z^2 = -z^2$, so $z^2 = -z^2$ $2z^2 = 0 $, therefore $z^2 = 0$ and $z=0$.
- Calculate $i^{-57}$
$i^{-57} = \frac{1}{i^{57}} = \frac{1}{i^{56} * i} = \frac{1}{(i^4)^{14} * i} = \frac{1}{1^{14} * i} = \frac{1}{i} = \frac{1}{i} * \frac{-i}{-i} = \frac{-i}{-i^2} = \frac{-i}{1} = -i$
- Find the complex number whose conjugate is $\frac{1}{i-1}$
If the conjugate of a complex number $z$ is $\frac{1}{i-1}$, then $z = \overline{(\frac{1}{i-1})} = \frac{1}{\overline{(i-1)}} = \frac{1}{-i-1} = \frac{1}{-(i+1)} = \frac{-1}{i+1}$.
- (b) $2i$
- (a) $\frac{a^2+b^2}{c^2+d^2}$
- (d) no value of x.
- (d) z = 0
- (a) -i
- (b) $\frac{-1}{i+1}$
More Information
Complex numbers are represented in the form a + bi, where a and b are real numbers, and i is the imaginary unit, defined as the square root of -1.
Tips
- When squaring a complex number like $(1+i)^2$ forgetting the cross term $2i$.
- Not rationalizing the denominator when dividing complex numbers.
- Confusing a complex number with its conjugate. The conjugate of $a + bi$ is $a - bi$.
- Not knowing the powers of $i$: $i^1 = i$, $i^2 = -1$, $i^3 = -i$, $i^4 = 1$.
- When x+iy = sqrt((a+ib)/(c+id)), forgetting to take square of both sides before finding the magnitude.
AI-generated content may contain errors. Please verify critical information