Solve for x: log₄(x) = -2.5
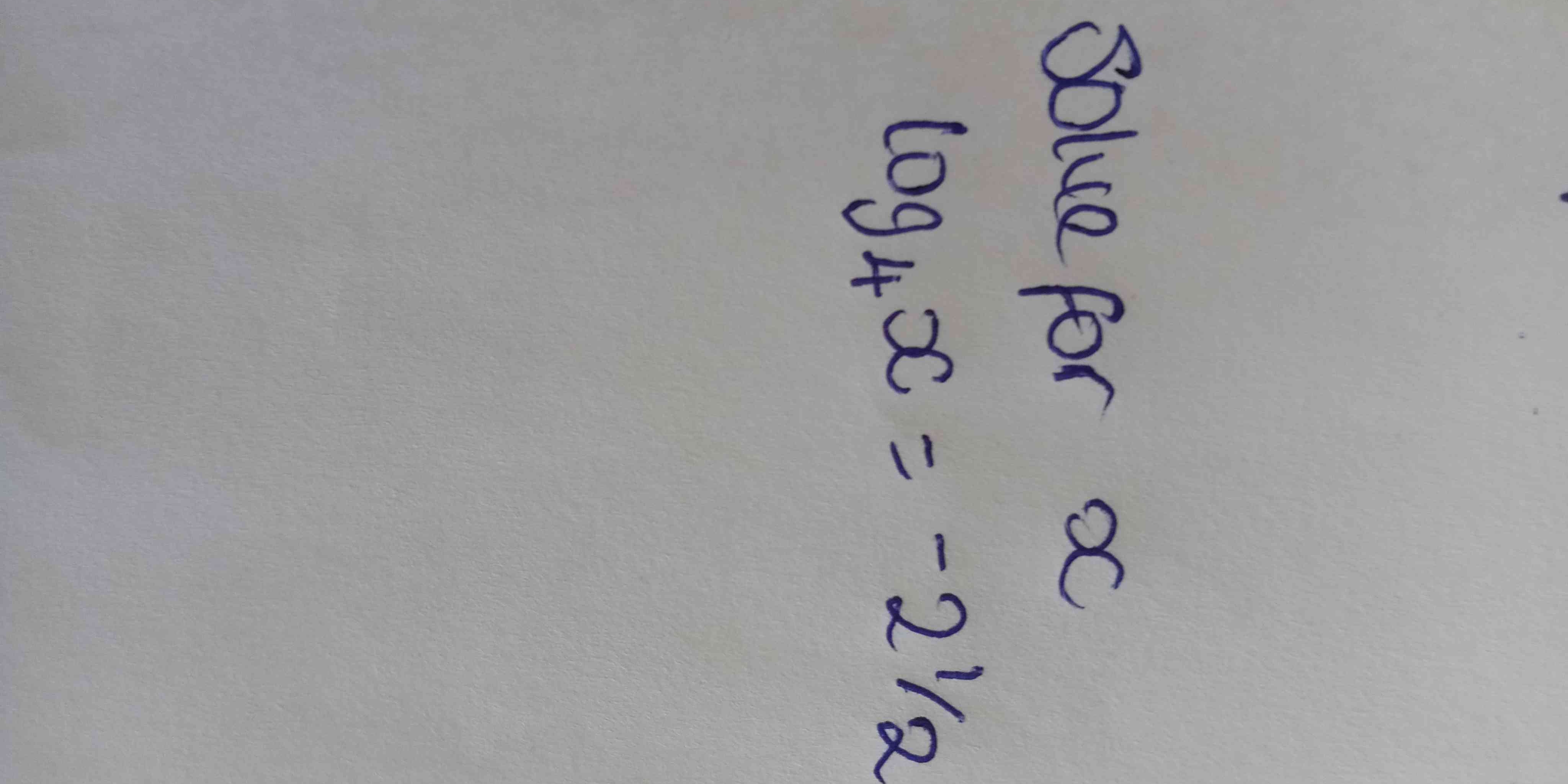
Understand the Problem
The question asks us to solve for x in the logarithmic equation log₄(x) = -2.5. We need to rewrite the equation using the properties of logarithms to isolate x and determine its value.
Answer
$x = \frac{1}{32}$
Answer for screen readers
$x = \frac{1}{32}$
Steps to Solve
- Rewrite the mixed number as a decimal
Convert $-2 \frac{1}{2}$ to a decimal, which is $-2.5$. So the equation is:
$log_4{x} = -2.5$
- Convert the logarithmic equation to exponential form
Using the property that $log_b{a} = c$ is equivalent to $b^c = a$, rewrite the equation:
$x = 4^{-2.5}$
- Rewrite the exponent as a fraction
Rewrite $-2.5$ as $-\frac{5}{2}$ to get:
$x = 4^{-\frac{5}{2}}$
- Use the property of negative exponents
Apply the rule $a^{-n} = \frac{1}{a^n}$:
$x = \frac{1}{4^{\frac{5}{2}}}$
- Rewrite the fractional exponent as a root and power
Recognize that $a^{\frac{m}{n}} = (a^{\frac{1}{n}})^m = ( \sqrt[n]{a})^m$. Therefore: $4^{\frac{5}{2}} = (4^{\frac{1}{2}})^5 = (\sqrt{4})^5$
- Simplify the expression
Calculate $\sqrt{4} = 2$. Therefore:
$(\sqrt{4})^5 = 2^5 = 32$
- Substitute the simplified value and solve for x
$x = \frac{1}{32}$
$x = \frac{1}{32}$
More Information
The value of $x$ that satisfies the equation $log_4{x} = -2\frac{1}{2}$ is $\frac{1}{32}$. This means that 4 raised to the power of -2.5 equals $\frac{1}{32}$.
Tips
A common mistake is incorrectly converting the logarithmic equation to exponential form. Another mistake is mishandling the negative and fractional exponents during simplification. Make sure to apply the correct rules for exponents and roots.
AI-generated content may contain errors. Please verify critical information