Solve: $3\frac{2}{3} \times 3\frac{2}{5} = $?
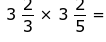
Understand the Problem
The question requires multiplying two mixed numbers together. We must first convert the mixed numbers into improper fractions, then multiply the fractions, and finally simplify the result if necessary.
Answer
$12\frac{7}{15}$
Answer for screen readers
$12\frac{7}{15}$
Steps to Solve
- Convert the first mixed number to an improper fraction
To convert $3\frac{2}{3}$ to an improper fraction, multiply the whole number (3) by the denominator (3) and add the numerator (2). Then, place the result over the original denominator.
$3\frac{2}{3} = \frac{(3 \times 3) + 2}{3} = \frac{9 + 2}{3} = \frac{11}{3}$
- Convert the second mixed number to an improper fraction
To convert $3\frac{2}{5}$ to an improper fraction, multiply the whole number (3) by the denominator (5) and add the numerator (2). Then, place the result over the original denominator.
$3\frac{2}{5} = \frac{(3 \times 5) + 2}{5} = \frac{15 + 2}{5} = \frac{17}{5}$
- Multiply the improper fractions
Multiply the numerators and multiply the denominators.
$\frac{11}{3} \times \frac{17}{5} = \frac{11 \times 17}{3 \times 5} = \frac{187}{15}$
- Convert the improper fraction to a mixed number
Divide the numerator (187) by the denominator (15). The quotient is the whole number part of the mixed number, the remainder is the numerator, and the denominator stays the same.
$187 \div 15 = 12$ with a remainder of $7$. So, $\frac{187}{15} = 12\frac{7}{15}$
$12\frac{7}{15}$
More Information
The result of multiplying $3\frac{2}{3}$ and $3\frac{2}{5}$ is $12\frac{7}{15}$. The fraction $\frac{7}{15}$ is already in its simplest form, as 7 and 15 have no common factors other than 1.
Tips
A common mistake is forgetting to convert the mixed numbers to improper fractions before multiplying. Another common mistake is incorrectly converting the mixed numbers to improper fractions, for example, by adding instead of multiplying. Finally, students might make arithmetic errors while multiplying or dividing, or they might forget to simplify the final fraction.
AI-generated content may contain errors. Please verify critical information