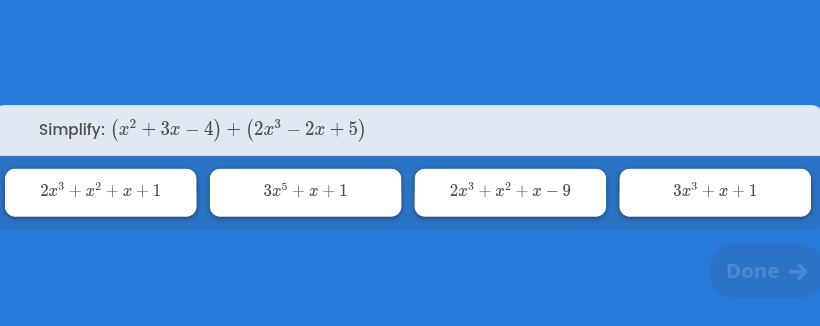
Simplify: $(x^2 + 3x - 4) + (2x^3 - 2x + 5)$
Understand the Problem
The question asks to simplify the sum of two polynomial expressions. We need to combine like terms from both expressions to produce a simplified polynomial.
Answer
$2x^3 + x^2 + x + 1$
Answer for screen readers
$2x^3 + x^2 + x + 1$
Steps to Solve
-
Write down the expression Write down the given expression: $(x^2 + 3x - 4) + (2x^3 - 2x + 5)$
-
Remove parentheses Since we are adding the polynomials, we can remove the parentheses without changing any signs: $x^2 + 3x - 4 + 2x^3 - 2x + 5$
-
Combine like terms Identify and combine terms with the same power of $x$:
- $x^2$ term: $x^2$ (only one $x^2$ term)
- $x$ terms: $3x - 2x = x$
- Constant terms: $-4 + 5 = 1$
- $x^3$ term: $2x^3$ (only one $x^3$ term)
- Write the simplified polynomial Combine the simplified terms, usually starting with the term with the highest power of $x$: $2x^3 + x^2 + x + 1$
$2x^3 + x^2 + x + 1$
More Information
Polynomial simplification is a fundamental concept in algebra. It involves combining like terms to reduce a polynomial to its simplest form.
Tips
A common mistake is to incorrectly combine unlike terms, for example, adding $x^2$ and $x$ terms together. Another common mistake is to not pay attention to the signs of the terms when removing parentheses or combining like terms. Remember to only combine terms that have the exact same variable and exponent.
AI-generated content may contain errors. Please verify critical information