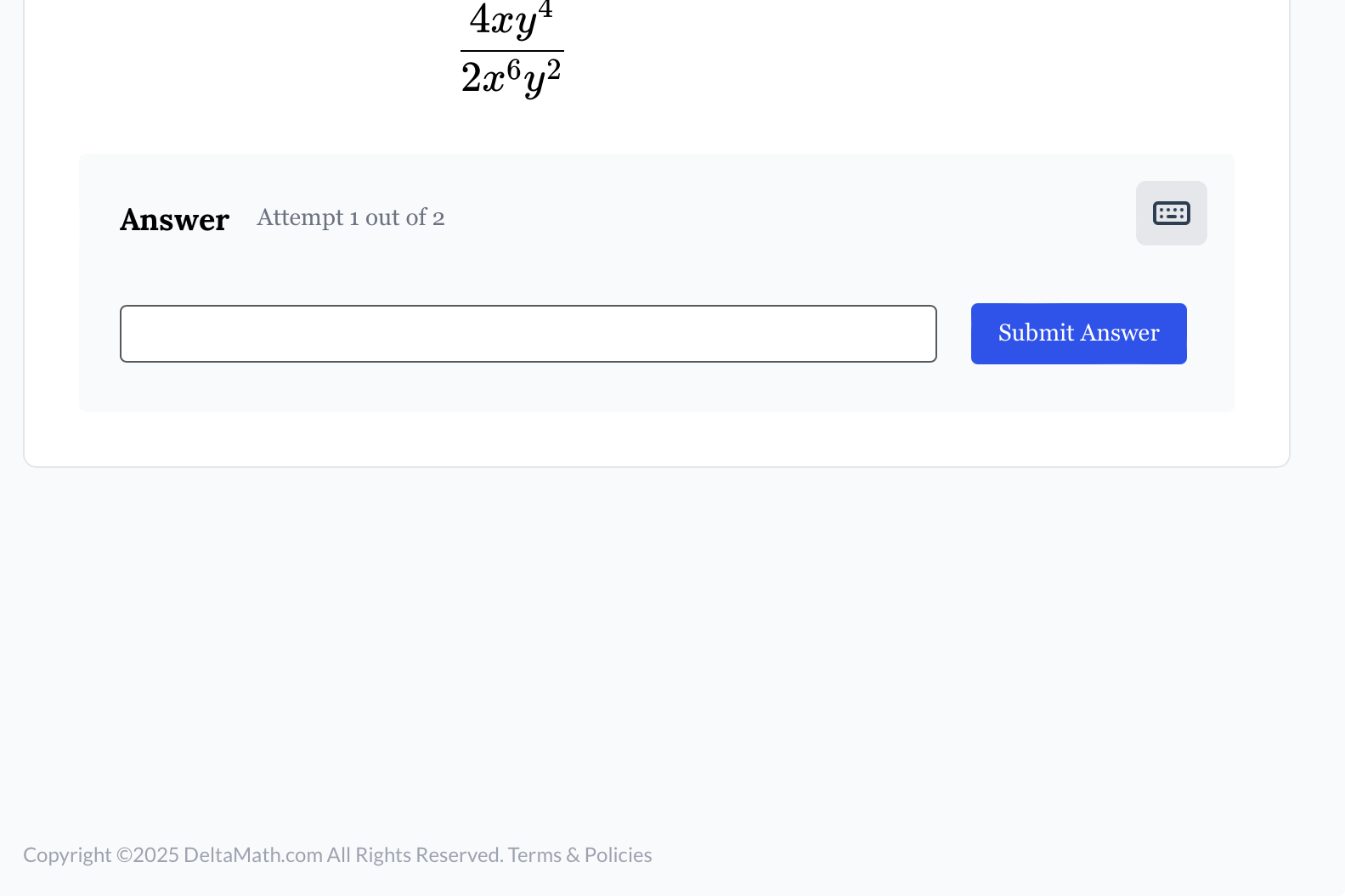
Simplify the expression 4xy^4 / 2x^6y^2.
Understand the Problem
The question is asking for the simplification of the expression given, which is 4xy^4 divided by 2x^6y^2. We will simplify the fraction by reducing both the coefficients and the variables.
Answer
The simplified expression is \( \frac{2y^2}{x^5} \).
Answer for screen readers
The simplified expression is ( \frac{2y^2}{x^5} ).
Steps to Solve
-
Simplify the Coefficient To simplify the coefficients, divide the numerator by the denominator: $$ \frac{4}{2} = 2 $$
-
Simplify the Variable $x$ In the numerator, we have $x^1$ and in the denominator, $x^6$. Using the rule of exponents $a^m / a^n = a^{m-n}$, we get: $$ \frac{x^1}{x^6} = x^{1-6} = x^{-5} $$
-
Simplify the Variable $y$ Next, simplify the variable $y$. In the numerator, we have $y^4$ and in the denominator, $y^2$. Again using the exponent rule: $$ \frac{y^4}{y^2} = y^{4-2} = y^2 $$
-
Combine the Results Now combine the simplified components: $$ 2 \cdot x^{-5} \cdot y^2 $$
-
Express with Positive Exponents To express $x^{-5}$ with a positive exponent, we write it as: $$ \frac{2y^2}{x^5} $$
The simplified expression is ( \frac{2y^2}{x^5} ).
More Information
This fraction represents a simplified version of the given expression. Simplifying fractions involves reducing both numeric and variable components.
Tips
- Forgetting to apply exponent rules correctly, especially when subtracting the exponents.
- Not simplifying the coefficients before addressing the variables.
AI-generated content may contain errors. Please verify critical information