Simplify \( \frac{3/7}{3} \\)
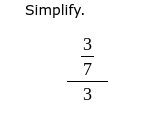
Understand the Problem
The question is asking to simplify the expression \( \frac{3/7}{3} \. This involves working with fractions and division.
Answer
The simplified expression is \( \frac{1}{7} \).
Answer for screen readers
The simplified expression is ( \frac{1}{7} ).
Steps to Solve
-
Rewrite the Expression We start by rewriting the division of a fraction by a whole number. The expression can be rewritten as: $$ \frac{3/7}{3} = \frac{3}{7} \div 3 $$
-
Convert the Division to Multiplication Next, we convert the division into multiplication by using the reciprocal of the whole number: $$ \frac{3}{7} \div 3 = \frac{3}{7} \times \frac{1}{3} $$
-
Multiply the Fractions Now, we multiply the fractions. To do this, multiply the numerators and the denominators: $$ \frac{3 \times 1}{7 \times 3} = \frac{3}{21} $$
-
Simplify the Resulting Fraction Finally, simplify the fraction ( \frac{3}{21} ) by dividing both the numerator and the denominator by their greatest common divisor, which is 3: $$ \frac{3 \div 3}{21 \div 3} = \frac{1}{7} $$
The simplified expression is ( \frac{1}{7} ).
More Information
When simplifying fractions involving division by whole numbers, remember that dividing by a number is the same as multiplying by its reciprocal. This helps in transforming the problem into a more manageable form.
Tips
- Confusing the operation between division and multiplication; always remember to convert division by a number into multiplication by its reciprocal.
- Failing to simplify the final fraction; always check if the resulting fraction can be simplified further.
