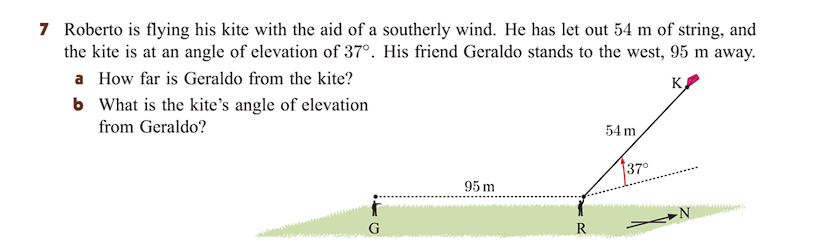
Roberto is flying his kite with the aid of a southerly wind. He has let out 54 m of string, and the kite is at an angle of elevation of 37°. His friend Geraldo stands to the west,... Roberto is flying his kite with the aid of a southerly wind. He has let out 54 m of string, and the kite is at an angle of elevation of 37°. His friend Geraldo stands to the west, 95 m away. a How far is Geraldo from the kite? b What is the kite's angle of elevation from Geraldo?
Understand the Problem
The problem describes a scenario where Roberto is flying a kite, and Geraldo is standing some distance away. We are asked to find the distance between Geraldo and the kite, and then find the kite's angle of elevation from Geraldo's perspective. This may require the use of trigonometric functions and the law of cosines.
Answer
a) $61.2 \text{ m}$ b) $32.1^\circ$
Answer for screen readers
a) The distance from Geraldo to the kite is approximately $61.2$ meters. b) The kite's angle of elevation from Geraldo is approximately $32.1^\circ$.
Steps to Solve
- Find the height of the kite
We can model the height $h$ of the kite using trigonometry. $$ h = 54 \sin(37^\circ) $$ $$ h \approx 32.5 \text{ m} $$
- Find the horizontal distance from Roberto to the point directly below the kite
The horizontal distance $x$ from Roberto to the point directly below the kite can be found using: $$ x = 54 \cos(37^\circ) $$ $$ x \approx 43.1 \text{ m} $$
- Find the horizontal distance from Geraldo to the point directly below the kite
The horizontal distance $d$ from Geraldo to the point directly below the kite can be calculated as: $$ d = 95 - x = 95 - 43.1 $$ $$ d \approx 51.9 \text{ m} $$
- Calculate the distance from Geraldo to the kite
Now, we can find the direct distance $D$ from Geraldo to the kite using the Pythagorean theorem: $$ D = \sqrt{d^2 + h^2} = \sqrt{51.9^2 + 32.5^2} $$ $$ D = \sqrt{2693.61 + 1056.25} = \sqrt{3749.86} $$ $$ D \approx 61.2 \text{ m} $$
- Calculate the angle of elevation from Geraldo to the kite
The angle of elevation $\theta$ from Geraldo to the kite can be calculated using: $$ \tan(\theta) = \frac{h}{d} = \frac{32.5}{51.9} $$ $$ \theta = \arctan\left(\frac{32.5}{51.9}\right) $$ $$ \theta \approx 32.1^\circ $$
a) The distance from Geraldo to the kite is approximately $61.2$ meters. b) The kite's angle of elevation from Geraldo is approximately $32.1^\circ$.
More Information
The problem involves using trigonometry and the Pythagorean theorem to find distances and angles in a 3D scenario.
Tips
A common mistake is forgetting to find the horizontal distance from Geraldo to the point directly under the kite. Instead, students might use the distance between Roberto and Geraldo directly, which is incorrect. Another common mistake is using the wrong trigonometric function (e.g., using cosine instead of sine for finding the height).
AI-generated content may contain errors. Please verify critical information