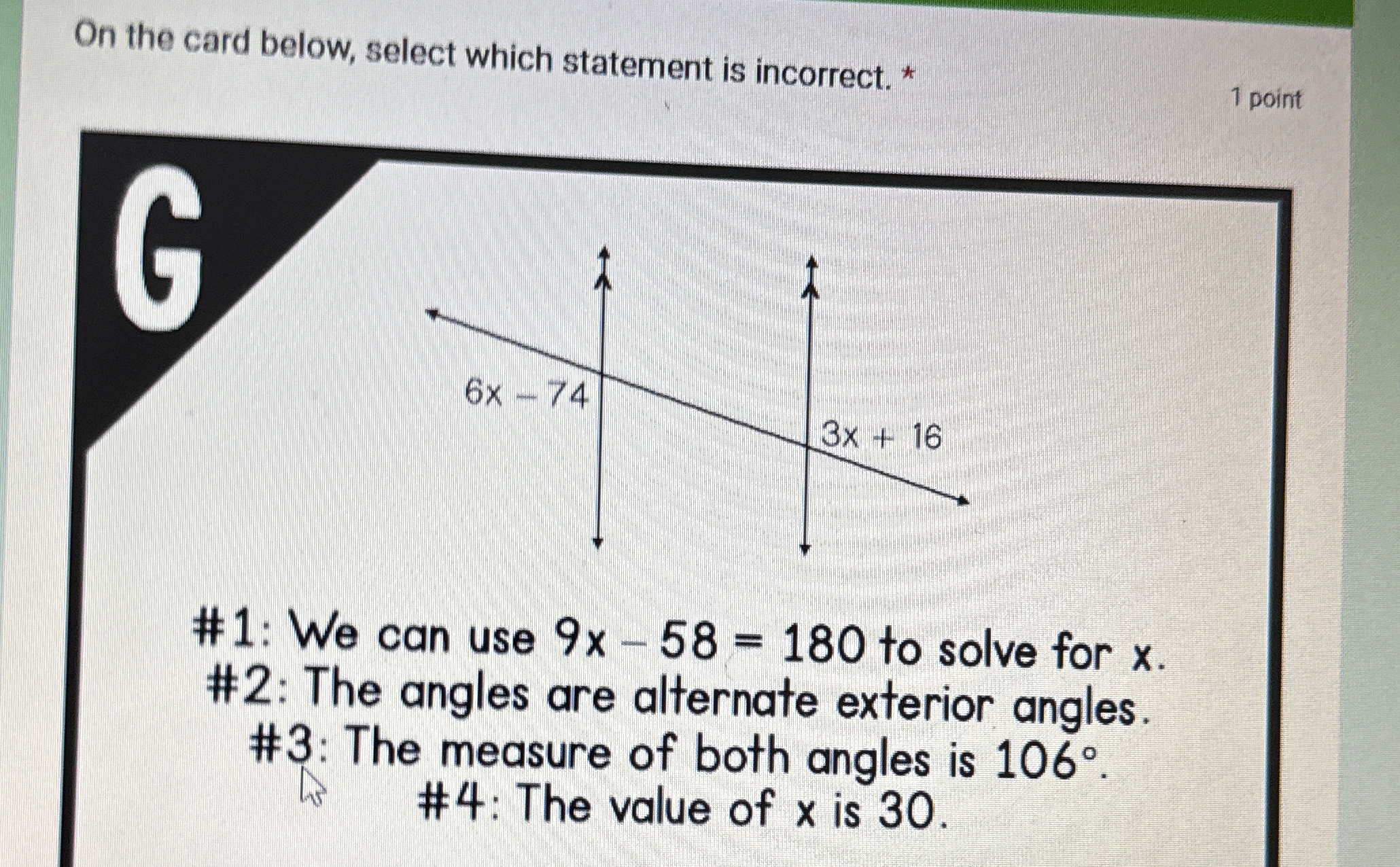
On the card below, select which statement is incorrect: #1: We can use 9x - 58 = 180 to solve for x. #2: The angles are alternate exterior angles. #3: The measure of both angles is... On the card below, select which statement is incorrect: #1: We can use 9x - 58 = 180 to solve for x. #2: The angles are alternate exterior angles. #3: The measure of both angles is 106°. #4: The value of x is 30. Read more

Steps to Solve

1. Identifying Angle Relationships

The two angles in the given diagram are represented as $6x - 74$ and $3x + 16$. Since these angles are created by parallel lines, they are corresponding angles, which means they are equal.

2. Setting Up the Equation

To solve for $x$, set the two angle expressions equal to each other:

$$ 6x - 74 = 3x + 16 $$

3. Solving for x

Subtract $3x$ from both sides to isolate terms involving $x$:

$$ 6x - 3x - 74 = 16 $$

This simplifies to:

$$ 3x - 74 = 16 $$

Next, add $74$ to both sides:

$$ 3x = 16 + 74 $$

Which simplifies to:

$$ 3x = 90 $$

Now, divide both sides by $3$ to find $x$:

$$ x = \frac{90}{3} $$

This results in:

$$ x = 30 $$

4. Finding the Measure of the Angles

Now substitute $x = 30$ back into the angle expressions to check their measures:

For the first angle:

$$ 6(30) - 74 = 180 - 74 = 106 $$

For the second angle:

$$ 3(30) + 16 = 90 + 16 = 106 $$

Both angles measure $106^\circ$, confirming they are indeed equal.

5. Evaluating the Statements

• Statement 1: True, as we set $6x - 74 = 3x + 16$.
• Statement 2: False; the angles are corresponding, not alternate exterior.
• Statement 3: True, both angles measure $106^\circ$.
• Statement 4: True, we've found $x = 30$.

Therefore, Statement 2 is incorrect.