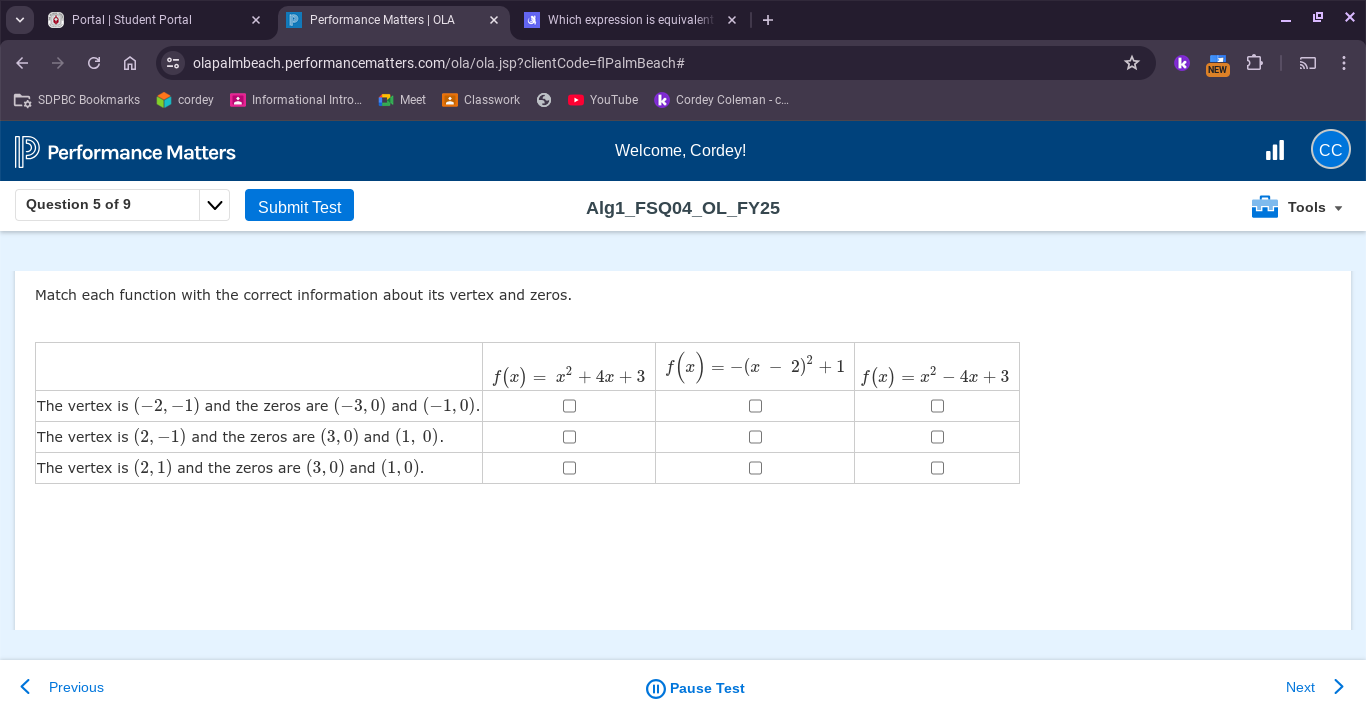
Match each function with the correct information about its vertex and zeros. The functions are: 1. f(x) = x² + 4x + 3 2. f(x) = -(x - 2)² + 1 3. f(x) = x² - 4x + 3 The verte... Match each function with the correct information about its vertex and zeros. The functions are: 1. f(x) = x² + 4x + 3 2. f(x) = -(x - 2)² + 1 3. f(x) = x² - 4x + 3 The vertex and zero options are: 1. The vertex is (-2, -1) and the zeros are (-3, 0) and (-1, 0). 2. The vertex is (2, -1) and the zeros are (3, 0) and (1, 0). 3. The vertex is (2, 1) and the zeros are (3, 0) and (1, 0).
Understand the Problem
The question requires matching each given quadratic function with the correct information about its vertex and zeros. This involves finding the vertex and zeros (roots) of each function and then matching them to the corresponding descriptions.
Answer
- $f(x) = x^2 + 4x + 3$: The vertex is $(-2,-1)$ and the zeros are $(-3, 0)$ and $(-1,0)$. - $f(x) = -(x - 2)^2 + 1$: The vertex is $(2, 1)$ and the zeros are $(3,0)$ and $(1,0)$. - $f(x) = x^2 - 4x + 3$: The vertex is $(2,-1)$ and the zeros are $(3,0)$ and $(1, 0)$.
Answer for screen readers
- $f(x) = x^2 + 4x + 3$: The vertex is (-2,-1) and the zeros are (-3, 0) and (-1,0).
- $f(x) = -(x - 2)^2 + 1$: The vertex is (2, 1) and the zeros are (3,0) and (1,0).
- $f(x) = x^2 - 4x + 3$: The vertex is (2,-1) and the zeros are (3,0) and (1, 0).
Steps to Solve
-
Analyze the first quadratic function $f(x) = x^2 + 4x + 3$
- Find the vertex: The x-coordinate of the vertex is given by $x = -\frac{b}{2a}$, where $a = 1$ and $b = 4$.
- $x = -\frac{4}{2(1)} = -2$. The y-coordinate is $f(-2) = (-2)^2 + 4(-2) + 3 = 4 - 8 + 3 = -1$. Thus, the vertex is $(-2, -1)$.
- Find the zeros: Solve $x^2 + 4x + 3 = 0$. Factoring gives $(x + 3)(x + 1) = 0$. Therefore, the zeros are $x = -3$ and $x = -1$. The zeros are $(-3, 0)$ and $(-1, 0)$.
- Match: This matches "The vertex is (-2, -1) and the zeros are (-3, 0) and (-1, 0)."
-
Analyze the second quadratic function $f(x) = -(x - 2)^2 + 1$
- Find the vertex: The function is in vertex form $f(x) = a(x - h)^2 + k$, where the vertex is $(h, k)$. In this case, $h = 2$ and $k = 1$. Thus, the vertex is $(2, 1)$.
- Find the zeros: Solve $-(x - 2)^2 + 1 = 0$.
- $(x - 2)^2 = 1$
- $x - 2 = \pm 1$
- $x = 2 \pm 1$, so $x = 3$ or $x = 1$. The zeros are $(3, 0)$ and $(1, 0)$.
- Match: This matches "The vertex is (2, 1) and the zeros are (3, 0) and (1, 0)."
-
Analyze the third quadratic function $f(x) = x^2 - 4x + 3$
- Find the vertex: The x-coordinate of the vertex is given by $x = -\frac{b}{2a}$, where $a = 1$ and $b = -4$.
- $x = -\frac{-4}{2(1)} = 2$. The y-coordinate is $f(2) = (2)^2 - 4(2) + 3 = 4 - 8 + 3 = -1$. Thus, the vertex is $(2, -1)$.
- Find the zeros: Solve $x^2 - 4x + 3 = 0$. Factoring gives $(x - 3)(x - 1) = 0$. Therefore, the zeros are $x = 3$ and $x = 1$. The zeros are $(3, 0)$ and $(1, 0)$.
- Match: This matches "The vertex is (2, -1) and the zeros are (3, 0) and (1, 0)."
- $f(x) = x^2 + 4x + 3$: The vertex is (-2,-1) and the zeros are (-3, 0) and (-1,0).
- $f(x) = -(x - 2)^2 + 1$: The vertex is (2, 1) and the zeros are (3,0) and (1,0).
- $f(x) = x^2 - 4x + 3$: The vertex is (2,-1) and the zeros are (3,0) and (1, 0).
More Information
Quadratic functions can be expressed in different forms, each providing insights into the function's characteristics:
- Standard Form: $f(x) = ax^2 + bx + c$
- Vertex Form: $f(x) = a(x - h)^2 + k$, where $(h, k)$ is the vertex.
- Factored Form: $f(x) = a(x - r_1)(x - r_2)$, where $r_1$ and $r_2$ are the roots or zeros.
Tips
A common mistake is incorrectly calculating the vertex coordinates or factoring the quadratic equation to find the zeros. Another is confusing the signs when using the vertex form $f(x) = a(x - h)^2 + k$. Paying close attention to detail and double-checking calculations can help avoid these mistakes.
AI-generated content may contain errors. Please verify critical information