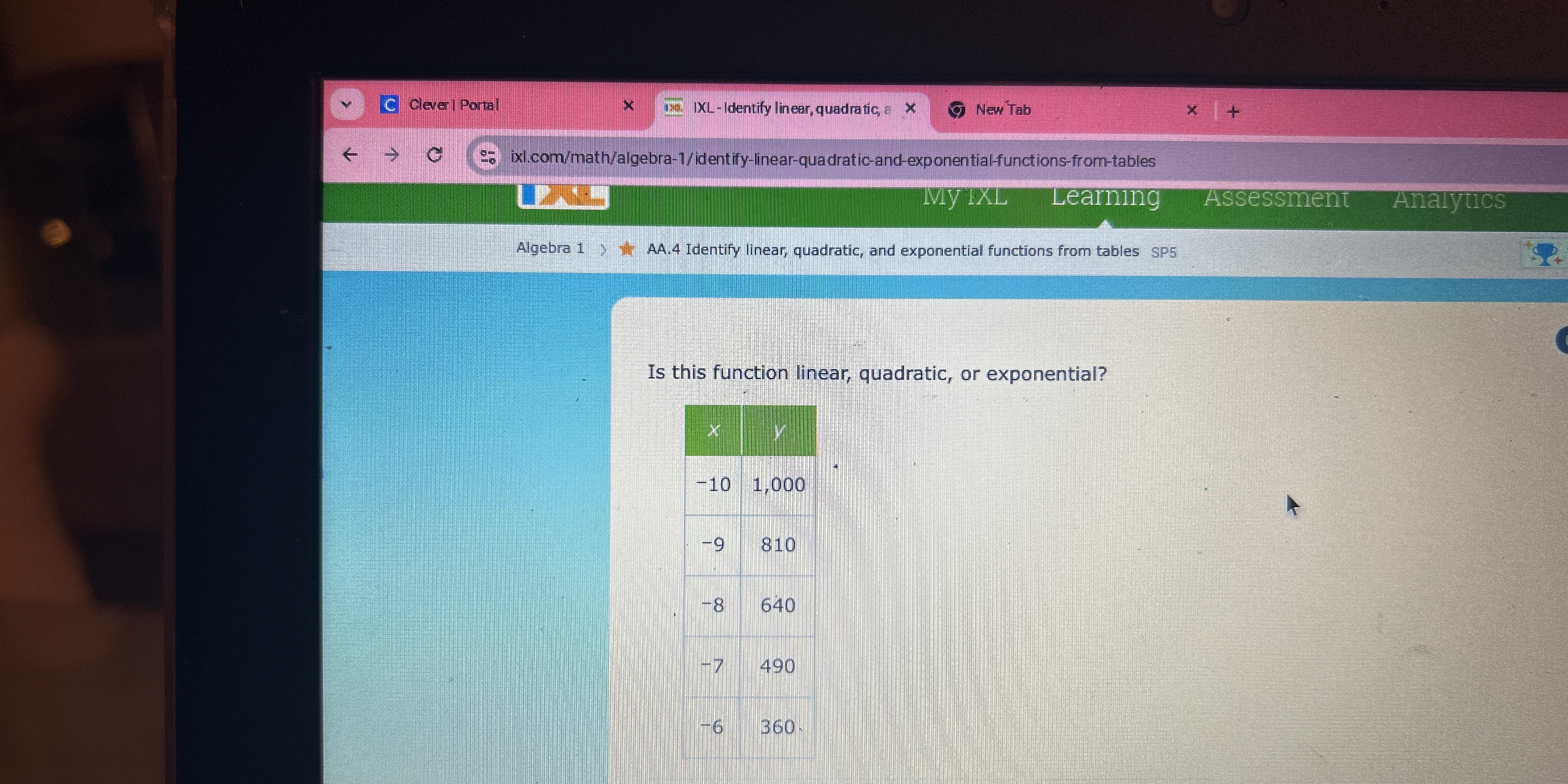
Is this function linear, quadratic, or exponential given the following values? x = -10, y = 1000 x = -9, y = 810 x = -8, y = 640 x = -7, y = 490 x = -6, y = 360
Understand the Problem
The question is asking to identify the type of function (linear, quadratic, or exponential) given a table of x and y values. To solve this, we need to analyze the differences (or ratios) between consecutive y-values to determine the pattern and thus identify the function type. This looks like a homework question related to Algebra 1.
Answer
Quadratic
Answer for screen readers
Quadratic
Steps to Solve
- Calculate the first differences in y-values
Subtract consecutive y-values to see if there's a constant difference.
$810 - 1000 = -190$ $640 - 810 = -170$ $490 - 640 = -150$ $360 - 490 = -130$
- Analyze the first differences
The first differences are not constant ($-190, -170, -150, -130$). So, the function is not linear.
- Calculate the second differences
Since the first differences are not constant, calculate the differences between the first differences.
$-170 - (-190) = 20$ $-150 - (-170) = 20$ $-130 - (-150) = 20$
- Analyze the second differences
The second differences are constant ($20$). This indicates that the function is quadratic.
Quadratic
More Information
Quadratic functions have a constant second difference when the x-values are evenly spaced.
Tips
A common mistake is to stop after calculating the first differences and incorrectly conclude the function is linear if the differences are close but not exactly the same. It's important to proceed to calculate second differences if the first differences are not constant. Also, students sometimes mix up first and second differences.
AI-generated content may contain errors. Please verify critical information