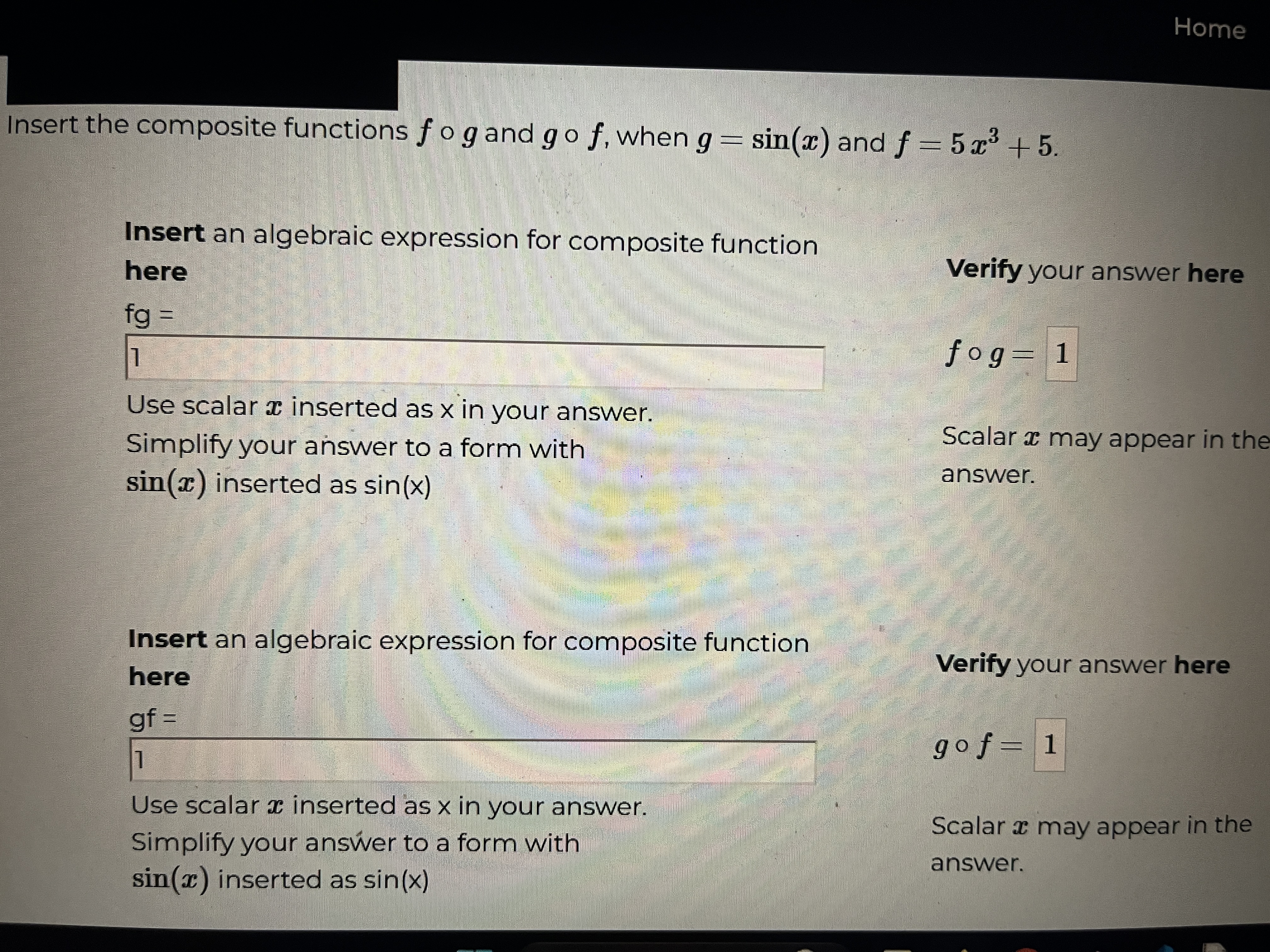
Insert the composite functions f(g) and g(f), when g = sin(x) and f = 5x^3 + 5. Insert an algebraic expression for composite function fg and gf, using scalar x and simplify your an... Insert the composite functions f(g) and g(f), when g = sin(x) and f = 5x^3 + 5. Insert an algebraic expression for composite function fg and gf, using scalar x and simplify your answers to include sin(x).
Understand the Problem
The question is asking to find and insert algebraic expressions for the composite functions f(g(x)) and g(f(x)) when g and f are given functions. It also requires simplifying the answers to include sin(x).
Answer
- For \( f(g(x)) \): \( 5\sin(x)^3 + 5 \) - For \( g(f(x)) \): \( \sin(5x^3 + 5) \)
Answer for screen readers
-
For ( f(g(x)) ): ( 5\sin(x)^3 + 5 )
-
For ( g(f(x)) ): ( \sin(5x^3 + 5) )
Steps to Solve
- Identify the Functions
We have two functions:
- ( g(x) = \sin(x) )
- ( f(x) = 5x^3 + 5 )
- Find ( f(g(x)) )
This means we need to substitute ( g(x) ) into ( f(x) ):
$$ f(g(x)) = f(\sin(x)) = 5(\sin(x))^3 + 5 $$
- Simplify ( f(g(x)) )
The expression is already simplified as ( 5\sin(x)^3 + 5 ), which includes ( \sin(x) ).
- Find ( g(f(x)) )
Now we substitute ( f(x) ) into ( g(x) ):
$$ g(f(x)) = g(5x^3 + 5) = \sin(5x^3 + 5) $$
- Simplify ( g(f(x)) )
The expression can't be simplified further, so we have:
$$ g(f(x)) = \sin(5x^3 + 5) $$
-
For ( f(g(x)) ): ( 5\sin(x)^3 + 5 )
-
For ( g(f(x)) ): ( \sin(5x^3 + 5) )
More Information
The composite function ( f(g(x)) ) shows how ( g(x) ), which gives the sine of ( x ), is used in ( f(x) ). Similarly, ( g(f(x)) ) applies the sine function to a cubic polynomial, revealing how composite functions can change the output significantly.
Tips
- Forgetting to substitute the inner function completely. Make sure to plug all parts of ( g(x) ) into ( f(x) ) and vice versa.
- Not simplifying fully. Always check to see if the expression can be simplified or rewritten using the required function form.
AI-generated content may contain errors. Please verify critical information