In a class of 80 students, 40 study Physics, 48 study Mathematics, and 44 study Chemistry. 20 study Physics and Mathematics, 24 study only two of the three subjects. If every stude... In a class of 80 students, 40 study Physics, 48 study Mathematics, and 44 study Chemistry. 20 study Physics and Mathematics, 24 study only two of the three subjects. If every student studies at least one of the three subjects, find: (a) the number of students who study all three subjects, (b) the number of students who study only Mathematics and Chemistry.
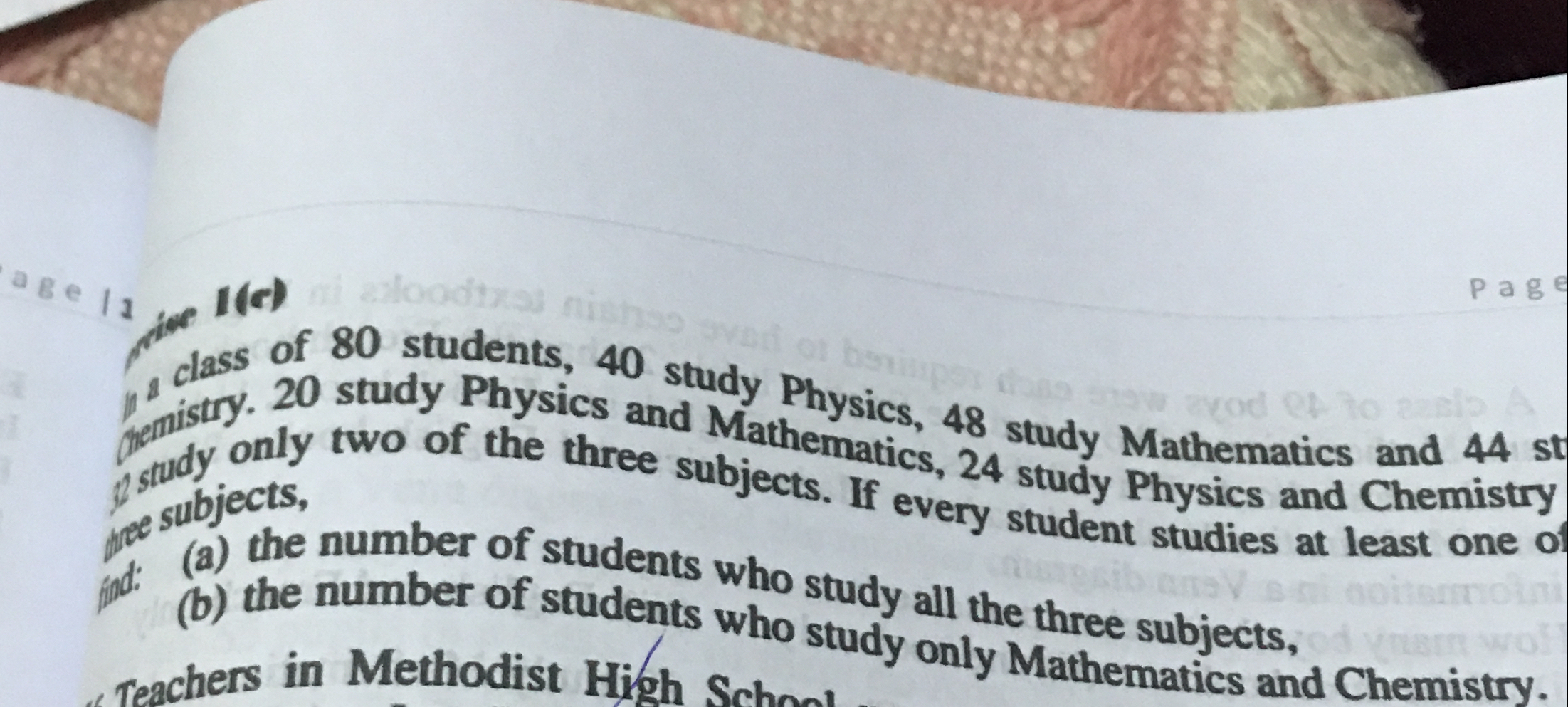
Understand the Problem
The question provides statistics about students studying three subjects: Physics, Mathematics, and Chemistry. It asks to find (a) the number of students who study all three subjects and (b) the number of students who study only Mathematics and Chemistry. This is a problem involving set theory, specifically Venn diagrams.
Answer
(a) 14, (b) 8
Answer for screen readers
(a) The number of students who study all three subjects is $14$.
(b) The number of students who study only Mathematics and Chemistry is $8$.
Steps to Solve
- Identify the given data
We have the following information from the problem:
- Total students, $T = 80$
- Students studying Physics, $P = 40$
- Students studying Mathematics, $M = 48$
- Students studying Chemistry, $C = 44$
- Students studying Physics and Mathematics, $PM = 20$
- Students studying Physics and Chemistry, $PC = 24$
- Students studying Mathematics and Chemistry, $MC = 22$
- Students studying all three subjects, $x$ (to be determined)
-
Set up the equation for students studying at least one subject
Since every student studies at least one subject, we can use the inclusion-exclusion principle:
$$ T = P + M + C - (PM + PC + MC) + x $$
Substituting the known values:
$$ 80 = 40 + 48 + 44 - (20 + 24 + 22) + x $$ -
Simplify the equation
Calculating the right side:
$$ 80 = 40 + 48 + 44 - 66 + x $$
Simplifying:
$$ 80 = 66 + x $$
Now rearranging gives us:
$$ x = 80 - 66 $$ $$ x = 14 $$
Thus, the number of students studying all three subjects is 14.
- Find students studying only Mathematics and Chemistry
Let $y$ represent the students studying only Mathematics and Chemistry.
Using the information provided, we can express those studying only Mathematics and Chemistry with respect to $x$ (students studying all three subjects) as:
$$ MC = y + x $$
where $MC$ is the number of students studying Mathematics and Chemistry, which we know is 22.
Substituting the known values:
$$ 22 = y + 14 $$
Solving for $y$:
$$ y = 22 - 14 $$
$$ y = 8 $$
Thus, the number of students studying only Mathematics and Chemistry is 8.
(a) The number of students who study all three subjects is $14$.
(b) The number of students who study only Mathematics and Chemistry is $8$.
More Information
This problem involves basic principles of set theory, specifically the use of the inclusion-exclusion principle to account for overlapping sets. Venn diagrams can also be useful for visualizing relationships between the subjects.
Tips
- Misapplying the inclusion-exclusion principle by not carefully accounting for overlaps.
- Forgetting to simplify the equations properly, which can lead to incorrect results.
- Confusing students studying two subjects with those studying only two subjects.
AI-generated content may contain errors. Please verify critical information