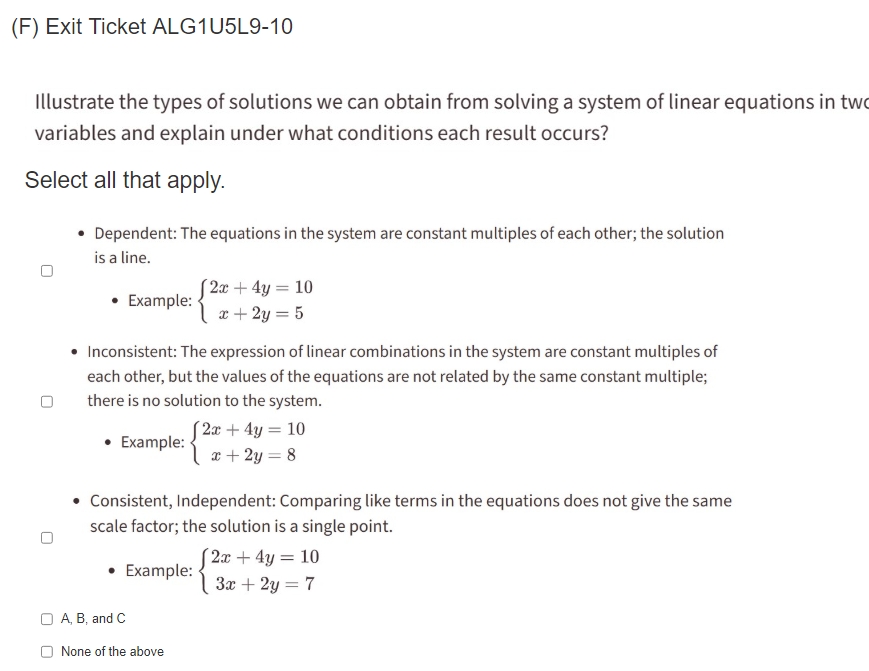
Illustrate the types of solutions we can obtain from solving a system of linear equations in two variables and explain under what conditions each result occurs. Select all that app... Illustrate the types of solutions we can obtain from solving a system of linear equations in two variables and explain under what conditions each result occurs. Select all that apply.
Understand the Problem
The question is asking to illustrate and explain the types of solutions that can be obtained from solving a system of linear equations in two variables. It seeks to categorize the solutions as dependent, inconsistent, or consistent independent, along with providing examples and the conditions under which each type occurs.
Answer
A, B, and C
Answer for screen readers
The answer is A, B, and C.
Steps to Solve
-
Define Dependent Systems A dependent system consists of equations that are multiples of one another. The lines represented by the equations coincide, meaning there are infinite solutions.
- For example, the equations: $$ 2x + 4y = 10 $$ and $$ x + 2y = 5 $$ are dependent because the second equation is half of the first.
-
Define Inconsistent Systems An inconsistent system has equations that do not intersect. They are parallel lines and have no common solutions.
- For example, the equations: $$ 2x + 4y = 10 $$ and $$ x + 2y = 8 $$ are inconsistent, as they result in two parallel lines.
-
Define Consistent Independent Systems A consistent independent system has unique solutions where lines intersect at a single point. They are not multiples of each other.
- For example, the equations: $$ 2x + 4y = 10 $$ and $$ 3x + 2y = 7 $$ intersect at one point, making them consistent and independent.
The answer is A, B, and C.
More Information
Each type of system of equations—dependent, inconsistent, and consistent independent—describes a different relationship between the equations. Understanding these types is fundamental in algebra, as they determine the nature of solutions in linear systems.
Tips
- Confusing dependent and independent systems by not checking if the coefficients are multiples.
- Misidentifying inconsistent systems as consistent if an intersection point is incorrectly presumed.
AI-generated content may contain errors. Please verify critical information