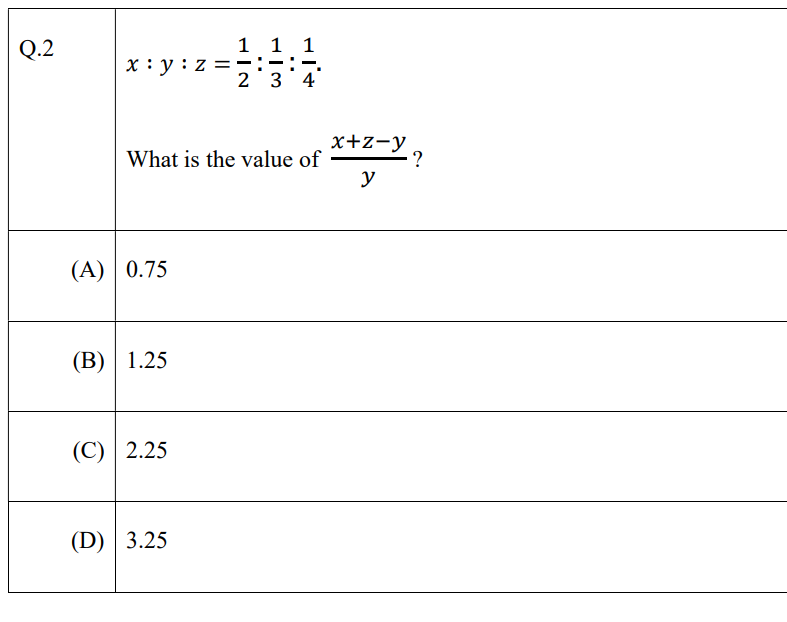
If x:y:z = 1/2 : 1/3 : 1/4, what is the value of (x+z-y)/y?

Steps to Solve

1. Find a common denominator for the ratio

To get rid of the fractions in the ratio $x:y:z = \frac{1}{2} : \frac{1}{3} : \frac{1}{4}$, we find the least common multiple (LCM) of the denominators 2, 3, and 4. The LCM is 12.

2. Multiply each term in the ratio by the common denominator

Multiply each fraction by 12: $x:y:z = (\frac{1}{2} \cdot 12) : (\frac{1}{3} \cdot 12) : (\frac{1}{4} \cdot 12) = 6:4:3$

3. Express $x$ and $z$ in terms of $y$

From the ratio $x:y:z = 6:4:3$, we can write $x = \frac{6}{4}y = \frac{3}{2}y$ and $z = \frac{3}{4}y$.

4. Substitute $x$ and $z$ in the expression

Now, substitute $x = \frac{3}{2}y$ and $z = \frac{3}{4}y$ into the expression $\frac{x+z-y}{y}$:

$\frac{x+z-y}{y} = \frac{\frac{3}{2}y + \frac{3}{4}y - y}{y}$

5. Simplify the numerator

Combine the terms in the numerator: $\frac{3}{2}y + \frac{3}{4}y - y = \frac{6}{4}y + \frac{3}{4}y - \frac{4}{4}y = \frac{6+3-4}{4}y = \frac{5}{4}y$

6. Simplify the entire expression

Substitute the simplified numerator back into the expression: $\frac{\frac{5}{4}y}{y} = \frac{5}{4}$

7. Convert the fraction to a decimal

Convert $\frac{5}{4}$ to a decimal: $\frac{5}{4} = 1.25$