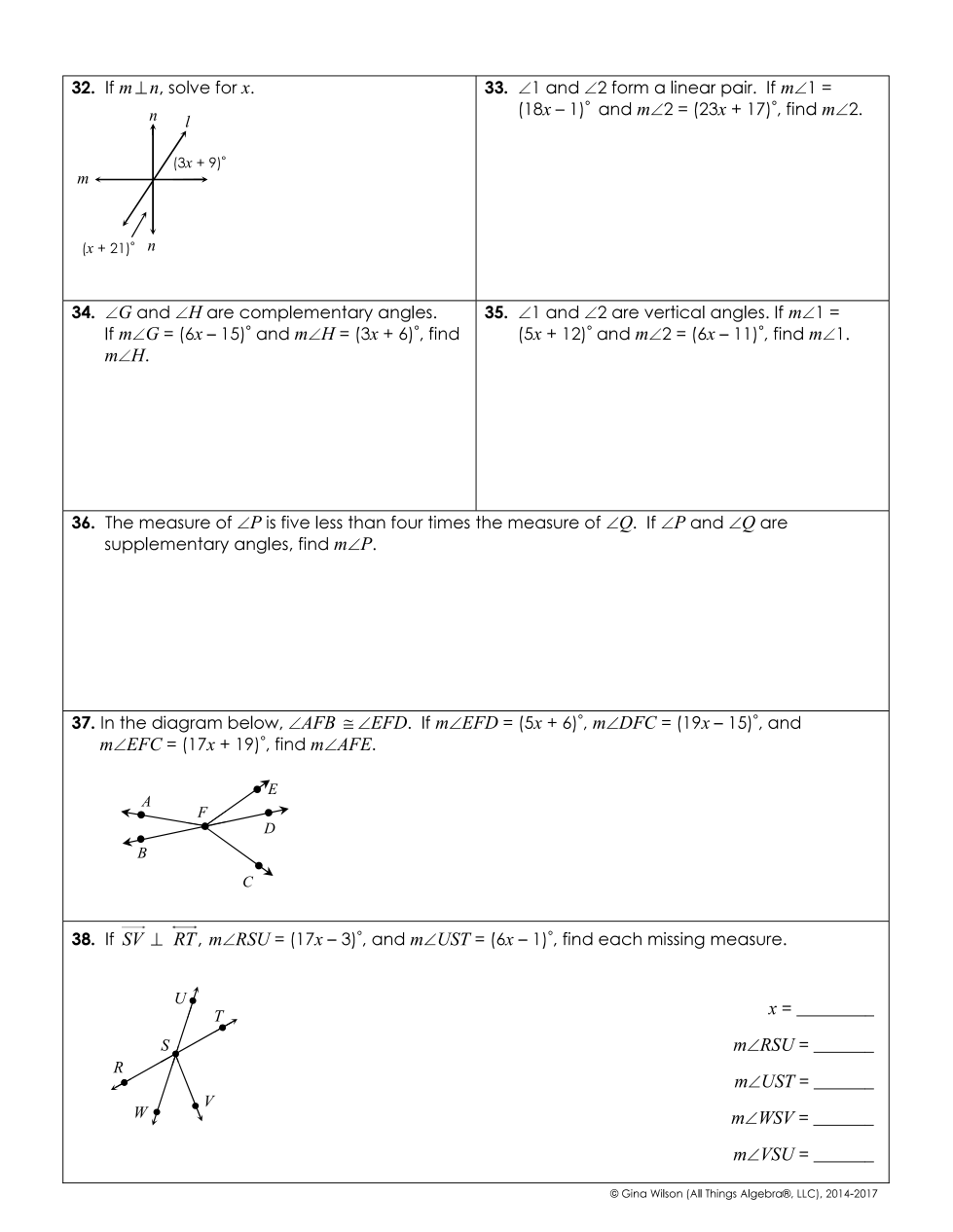
If m l n, solve for x. Angle 1 and Angle 2 form a linear pair. If m Angle 1 = (18x - 1)° and m Angle 2 = (23x + 17)°, find m Angle 2. Angle G and Angle H are complementary angles.... If m l n, solve for x. Angle 1 and Angle 2 form a linear pair. If m Angle 1 = (18x - 1)° and m Angle 2 = (23x + 17)°, find m Angle 2. Angle G and Angle H are complementary angles. If m Angle G = (6x - 15)° and m Angle H = (3x + 6)°, find m Angle H. Angle 1 and Angle 2 are vertical angles. If m Angle 1 = (5x + 12)° and m Angle 2 = (6x - 11)°, find m Angle 1. The measure of angle P is five less than four times the measure of angle Q. If angle P and angle Q are supplementary angles, find m Angle P. In the diagram below, Angle AFB ≅ Angle EFD. If m Angle EFD = (5x + 6)°, m Angle DFC = (19x - 15)°, and m Angle EFC = (17x + 19)°, find m Angle AFE. If SV ⊥ RT, m Angle RSU = (17x - 3)°, and m Angle UST = (6x - 1)°, find each missing measure. Read more

Steps to Solve

1. Linear Pair Angles (Problem 33)

Since angles 1 and 2 form a linear pair, their measures must add up to $180^\circ$.

Set up the equation: $$(18x - 1) + (23x + 17) = 180$$

2. Combine Like Terms

Combine the $x$ terms and the constant terms: $$18x + 23x - 1 + 17 = 180$$

This simplifies to: $$41x + 16 = 180$$

3. Isolate x

Subtract 16 from both sides to isolate the term with $x$: $$41x = 164$$

4. Solve for x

Divide by 41: $$x = 4$$

5. Finding Angle m∠2

Now substitute $x = 4$ back into the expression for $m∠2$: $$m∠2 = 23(4) + 17 = 92 + 17 = 109^\circ$$

6. Complementary Angles (Problem 34)

For angles G and H which are complementary, set up the equation: $$(6x - 15) + (3x + 6) = 90$$

7. Combine Like Terms

This simplifies to: $$9x - 9 = 90$$

8. Isolate x

Add 9 to both sides: $$9x = 99$$

9. Solve for x

Divide by 9: $$x = 11$$

10. Finding m∠H

Now substitute $x = 11$ into the expression for $m∠H$: $$m∠H = 3(11) + 6 = 33 + 6 = 39^\circ$$

11. Vertical Angles (Problem 35)

For angles 1 and 2 that are vertical angles: $$m∠1 = m∠2$$

Set the equations equal: $$(5x + 12) = (6x - 11)$$

12. Isolate x

Rearranging gives: $$12 + 11 = 6x - 5x$$

This simplifies to: $$23 = x$$

13. Finding m∠1

Now we substitute back into the equation for $m∠1$: $$m∠1 = 5(23) + 12 = 115 + 12 = 127^\circ$$

14. Finding m∠P in Problem 36

Using the relationship given: Let $m∠Q = y$. Then: $$m∠P = 4y - 5$$

Set up the equation for supplementary angles: $$m∠P + m∠Q = 180$$

Substituting gives the equation: $$(4y - 5) + y = 180$$

15. Combine Terms

This simplifies to: $$5y - 5 = 180$$

16. Isolate y

Add 5 to both sides: $$5y = 185$$

17. Solve for y

Dividing gives: $$y = 37$$

18. Finding m∠P

Find $m∠P$: $$m∠P = 4(37) - 5 = 148 - 5 = 143^\circ$$

19. Finding m∠AFE in Problem 37

Using the relationship of angles: Set $m∠AFE = z$. Since $\angle AFB = \angle EFD$, we set up: $$z + (5x + 6) = (19x - 15)$$

20. Combine Terms

This simplifies to: $$z + 5x + 6 = 19x - 15$$

21. Isolate z

Rearranging gives: $$z = 19x - 15 - 5x - 6$$

22. Solve for x (would need values)

Then substitute back to find values.