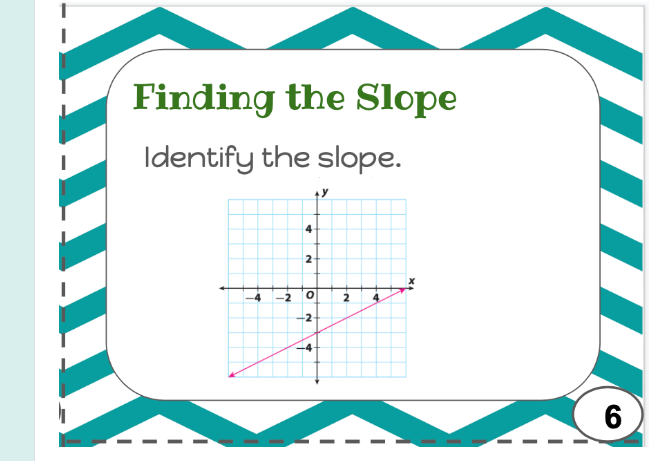
Identify the slope.
Understand the Problem
The question is asking us to identify the slope of the line shown in the coordinate graph. Slope is calculated by determining the rise over run between two points on the line.
Answer
The slope of the line is \( m = 1 \).
Answer for screen readers
The slope of the line is ( m = 1 ).
Steps to Solve
- Identify Two Points on the Line
From the graph, choose two clear points that the line passes through. Let's select the points ( (0, -2) ) and ( (4, 2) ).
- Calculate the Rise
The rise is the change in the ( y )-coordinates between the two points.
Using the points ( (0, -2) ) and ( (4, 2) ):
[ \text{Rise} = y_2 - y_1 = 2 - (-2) = 2 + 2 = 4 ]
- Calculate the Run
The run is the change in the ( x )-coordinates between the two points.
Using the same points:
[ \text{Run} = x_2 - x_1 = 4 - 0 = 4 ]
- Calculate the Slope
The slope ( m ) is calculated as the rise over run:
[ m = \frac{\text{Rise}}{\text{Run}} = \frac{4}{4} = 1 ]
The slope of the line is ( m = 1 ).
More Information
The slope measures how steep the line is and indicates that for every unit the line rises vertically, it also runs one unit horizontally. This means the line follows an upward trend from left to right, indicating a positive correlation.
Tips
- Confusing rise and run: Ensure to always subtract the ( y )-coordinates for rise and the ( x )-coordinates for run.
- Picking points that aren't on the line: Choose points that clearly lie on the line for accurate calculations.
AI-generated content may contain errors. Please verify critical information