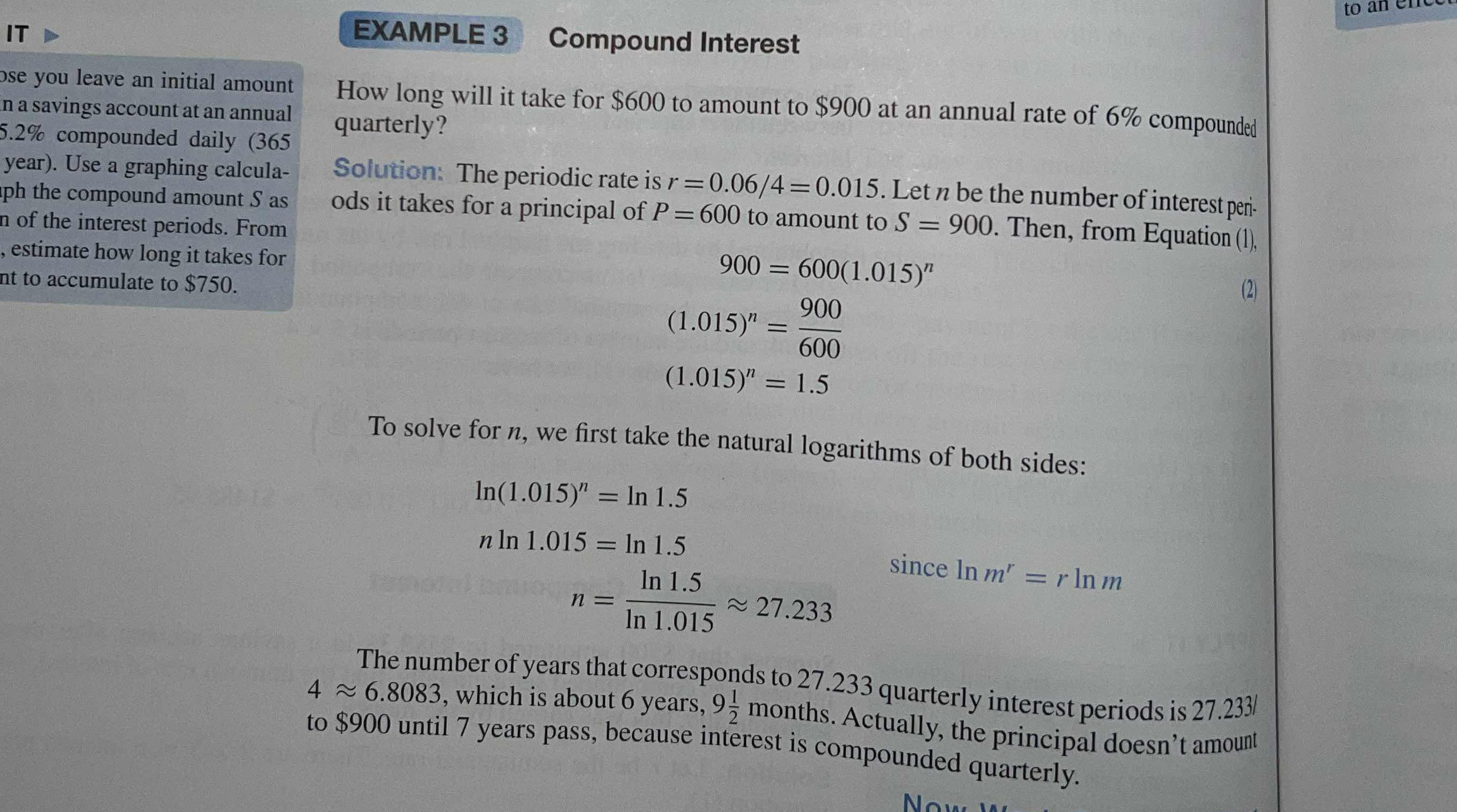
How long will it take for $600 to amount to $900 at an annual rate of 6% compounded quarterly?

Steps to Solve

1. Calculate the periodic interest rate The annual interest rate is 6%, and it's compounded quarterly, so we divide the annual rate by 4: $r = 0.06/4 = 0.015$

2. Set up the compound interest formula We want to find the number of periods, $n$, it takes for an initial investment of $P = 600$ to grow to $S = 900$. The compound interest formula is: $S = P(1 + r)^n$ In our case: $900 = 600(1 + 0.015)^n$

3. Isolate the exponential term Divide both sides by 600: $(1.015)^n = 900/600$ $(1.015)^n = 1.5$

4. Take the natural logarithm of both sides To solve for $n$, we take the natural logarithm (ln) of both sides: $ln(1.015)^n = ln(1.5)$

5. Use the logarithm power rule Apply the power rule of logarithms, which states that $ln(a^b) = b \cdot ln(a)$: $n \cdot ln(1.015) = ln(1.5)$

6. Solve for n Divide both sides by $ln(1.015)$: $n = \frac{ln(1.5)}{ln(1.015)}$

7. Calculate the value of n $n \approx 27.233$ This is the number of quarters.

8. Convert the number of quarters to years Divide the number of quarters by 4 to find the number of years: $Years = 27.233 / 4 \approx 6.8083$ years

9. Convert the decimal part of years into months $0.8083 * 12 \approx 9.7$ months. So approximately 6 years and 9.7 months are required