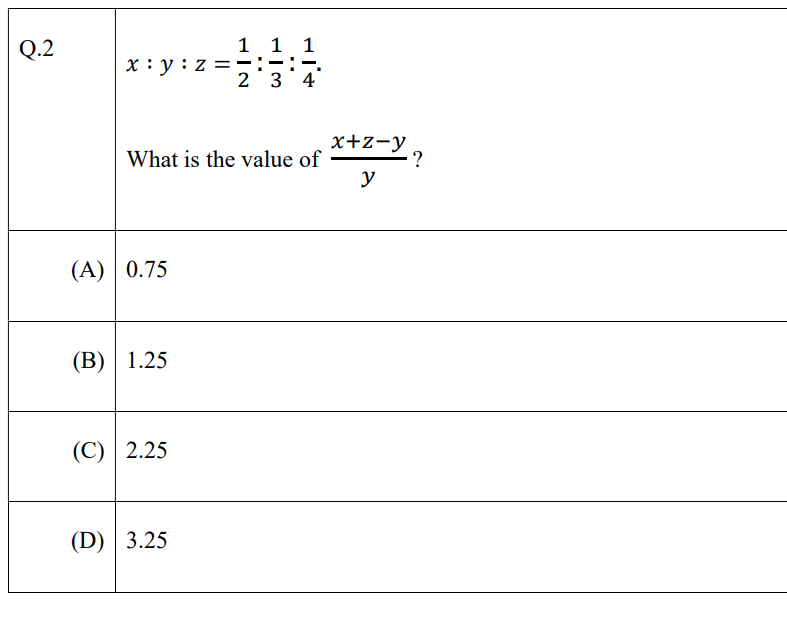
Given x:y:z = 1/2 : 1/3 : 1/4, what is the value of (x+z-y)/y?

Steps to Solve

1. Express the ratio with integers Multiply each fraction in the ratio $x:y:z = \frac{1}{2} : \frac{1}{3} : \frac{1}{4}$ by the least common multiple (LCM) of the denominators (2, 3, and 4), which is 12. This gives us: $$ x:y:z = \frac{12}{2} : \frac{12}{3} : \frac{12}{4} = 6:4:3 $$

2. Express x, y, and z with a common variable Let's express $x$, $y$, and $z$ in terms of a common variable, say $k$. From the ratio $6:4:3$, we have: $x = 6k$, $y = 4k$, and $z = 3k$.

3. Substitute into the expression Substitute these expressions into the given expression $\frac{x+z-y}{y}$: $$ \frac{x+z-y}{y} = \frac{6k + 3k - 4k}{4k} $$

4. Simplify the expression Simplify the numerator: $$ \frac{9k - 4k}{4k} = \frac{5k}{4k} $$ Now, cancel out the common factor $k$ (assuming $k \ne 0$): $$ \frac{5}{4} $$

5. Convert to decimal Convert the fraction to a decimal: $$ \frac{5}{4} = 1.25 $$