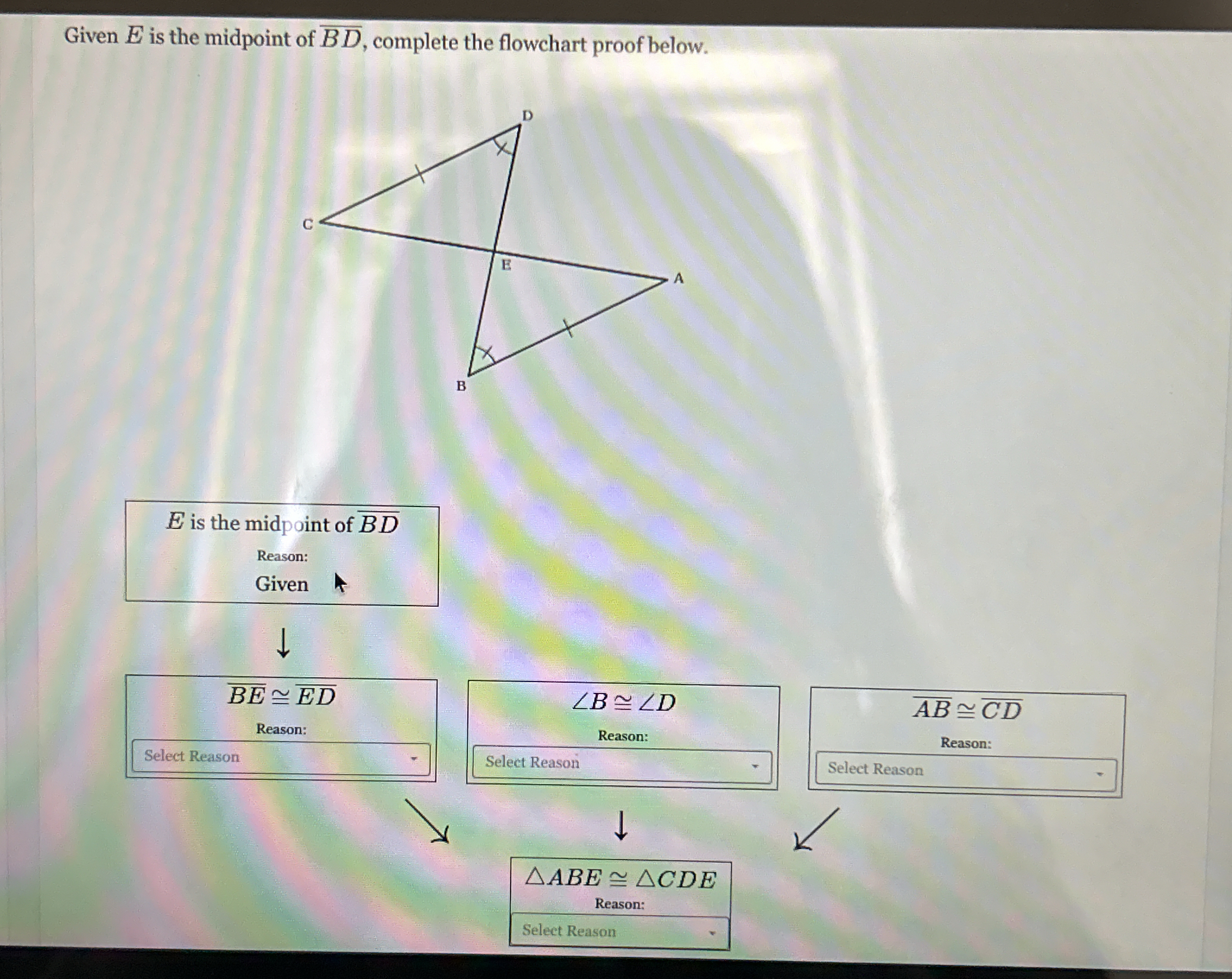
Given E is the midpoint of BD, complete the flowchart proof.
Understand the Problem
The question asks us to complete a flowchart proof given that point E is the midpoint of line segment BD. This involves filling in the reasons for each step in the proof to demonstrate that triangle ABE is congruent to triangle CDE.
Answer
$\overline{BE} \cong \overline{ED}$: Definition of Midpoint $\angle B \cong \angle D$: All right angles are congruent $\overline{AB} \cong \overline{CD}$: Given $\triangle ABE \cong \triangle CDE$: Side-Angle-Side (SAS)
Answer for screen readers
$\overline{BE} \cong \overline{ED}$ Reason: Definition of Midpoint
$\angle B \cong \angle D$ Reason: All right angles are congruent
$\overline{AB} \cong \overline{CD}$ Reason: Given
$\triangle ABE \cong \triangle CDE$ Reason: Side-Angle-Side (SAS)
Steps to Solve
- Determine the reason $\overline{BE} \cong \overline{ED}$
Since E is the midpoint of $\overline{BD}$, by the definition of a midpoint, $\overline{BE}$ and $\overline{ED}$ are congruent
- Determine the reason $\angle B \cong \angle D$
The diagram shows that $\angle B$ and $\angle D$ are right angles. Since all right angles are congruent, $\angle B \cong \angle D$
- Determine the reason $\overline{AB} \cong \overline{CD}$
This is given in the diagram, corresponding sides are marked as congruent.
- Determine the reason $\triangle ABE \cong \triangle CDE$
We have $\angle B \cong \angle D$, $\overline{AB} \cong \overline{CD}$, and $\overline{BE} \cong \overline{ED}$. Thus, by Side-Angle-Side (SAS) congruence, $\triangle ABE \cong \triangle CDE$.
$\overline{BE} \cong \overline{ED}$ Reason: Definition of Midpoint
$\angle B \cong \angle D$ Reason: All right angles are congruent
$\overline{AB} \cong \overline{CD}$ Reason: Given
$\triangle ABE \cong \triangle CDE$ Reason: Side-Angle-Side (SAS)
More Information
The Side-Angle-Side (SAS) congruence postulate is a way to prove that two triangles are congruent. It states that if two sides and the included angle (the angle between those two sides) of one triangle are congruent to the corresponding two sides and included angle of another triangle, then the two triangles are congruent.
Tips
A common mistake is to confuse the Side-Angle-Side (SAS) congruence postulate with Angle-Side-Angle (ASA) or Side-Side-Side (SSS). It's important to ensure that the angle is included between the two sides for SAS to apply. Another common mistake is to not realize that all right angles are congruent.
AI-generated content may contain errors. Please verify critical information