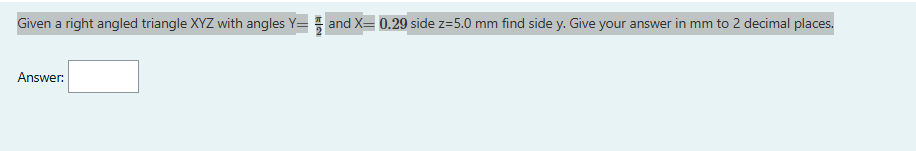
Given a right angled triangle XYZ with angles Y = π/2 and X = 0.29, side z = 5.0 mm. Find side y. Give your answer in mm to 2 decimal places.

Steps to Solve

1. Identify the angles and sides Given the angles in triangle XYZ:

• Angle $Y = \frac{\pi}{2}$ (90 degrees)
• Angle $X = 0.29$ radians

You can find angle $Z$ using the property that the sum of angles in a triangle is $\pi$ radians (or 180 degrees): $$ Z = \pi - (Y + X) = \pi - \left(\frac{\pi}{2} + 0.29\right) $$

2. Calculate angle Z Now, substitute the values: $$ Z = \pi - \left(\frac{\pi}{2} + 0.29\right) $$

This gives us: $$ Z \approx 2.851 - 0.29 \approx 2.561 $$ \text{ radians}

3. Use the sine rule Since we want to find side $y$ opposite angle $Y$, we can apply the sine rule, which states: $$ \frac{y}{\sin(Y)} = \frac{z}{\sin(Z)} $$

Rearranging gives: $$ y = z \cdot \frac{\sin(Y)}{\sin(Z)} $$

4. Substitute values Substituting the known values into the equation:

• $z = 5.0 , \text{mm}$
• $Y = \frac{\pi}{2}$

Thus: $$ y = 5.0 \cdot \frac{\sin\left(\frac{\pi}{2}\right)}{\sin(2.561)} $$

5. Calculate y Now we can compute: $$ y = 5.0 \cdot \frac{1}{\sin(2.561)} $$

Using a calculator, find $\sin(2.561)$ and then compute $y$.